Text erkannt:
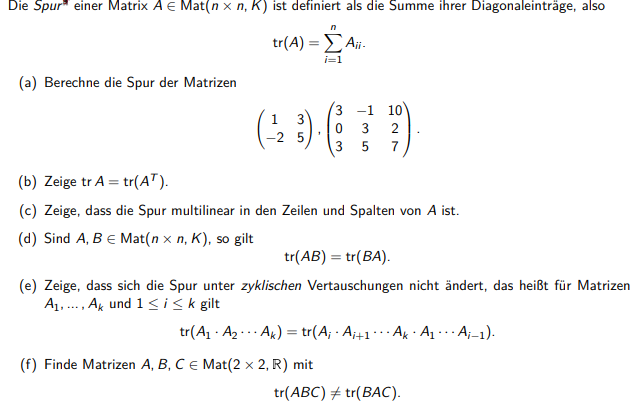
Die Spur \( ^{\text {r }} \) einer Matrix \( A \in \operatorname{Mat}(n \times n, K) \) ist definiert als die Summe ihrer Diagonaleinträge, also
$$ \operatorname{tr}(A)=\sum \limits_{i=1}^{n} A_{i i} $$
(a) Berechne die Spur der Matrizen
$$ \left(\begin{array}{cc} 1 & 3 \\ -2 & 5 \end{array}\right),\left(\begin{array}{ccc} 3 & -1 & 10 \\ 0 & 3 & 2 \\ 3 & 5 & 7 \end{array}\right) $$
(b) Zeige tr \( A=\operatorname{tr}\left(A^{T}\right) \).
(c) Zeige, dass die Spur multilinear in den Zeilen und Spalten von \( A \) ist.
(d) Sind \( A, B \in \operatorname{Mat}(n \times n, K) \), so gilt
$$ \operatorname{tr}(A B)=\operatorname{tr}(B A) $$
(e) Zeige, dass sich die Spur unter zyklischen Vertauschungen nicht ändert, das heißt für Matrizen \( A_{1}, \ldots, A_{k} \) und \( 1 \leq i \leq k \) gilt
$$ \operatorname{tr}\left(A_{1} \cdot A_{2} \cdots A_{k}\right)=\operatorname{tr}\left(A_{i} \cdot A_{i+1} \cdots A_{k} \cdot A_{1} \cdots A_{i-1}\right) $$
(f) Finde Matrizen \( A, B, C \in \operatorname{Mat}(2 \times 2, \mathbb{R}) \) mit
$$ \operatorname{tr}(A B C) \neq \operatorname{tr}(B A C) $$
Text erkannt:
Die Spur" einer Matrix \( A \in \operatorname{Mat}(n \times n, K) \) ist definiert als die Summe ihrer Diagonaleintrage, also
$$ \operatorname{tr}(A)=\sum \limits_{i=1}^{n} A_{i i} $$
(a) Berechne die Spur der Matrizen
$$ \left(\begin{array}{cc} 1 & 3 \\ -2 & 5 \end{array}\right),\left(\begin{array}{ccc} 3 & -1 & 10 \\ 0 & 3 & 2 \\ 3 & 5 & 7 \end{array}\right) $$
(b) Zeige tr \( A=\operatorname{tr}\left(A^{T}\right) \).
(c) Zeige, dass die Spur multilinear in den Zeilen und Spalten von \( A \) ist.
(d) Sind \( A, B \in \operatorname{Mat}(n \times n, K) \), so gilt
$$ \operatorname{tr}(A B)=\operatorname{tr}(B A) $$
(e) Zeige, dass sich die Spur unter zyklischen Vertauschungen nicht ändert, das heißt für Matrizen \( A_{1}, \ldots, A_{k} \) und \( 1 \leq i \leq k \) gilt
$$ \operatorname{tr}\left(A_{1} \cdot A_{2} \cdots A_{k}\right)=\operatorname{tr}\left(A_{i} \cdot A_{i+1} \cdots A_{k} \cdot A_{1} \cdots A_{i-1}\right) $$
(f) Finde Matrizen \( A, B, C \in \operatorname{Mat}(2 \times 2, \mathbb{R}) \) mit
$$ \operatorname{tr}(A B C) \neq \operatorname{tr}(B A C) $$
Aufgabe: