Schau hier bitte MAthe coach: Hier ist originale Aufgabe
Also meine Frage wie reche ich ...> diese marginal efficiency rate?
Das Gestzt unten ist Klar aber dann wie kommt er von 1 zu 2? ( Blau markiert)?
Möchte dir sagen ich bon chronisch krank und habe schwer KOneztration Problem und mache seite Jahren Ergpthrapie aber keine Verbesserung
Zuerst ich habe so gemacht: 1500 *2= 3000
dann 3000-700=2300
dann 2300 - 2300 *50%= 1150
Also 1150 und nicht 1650 wie im Buch steht. Ich danke ein Druckfehler. Aber darum geht es niauch das sollt 10000 und nicht 1000 , wei im Buch sthet
Text erkannt:
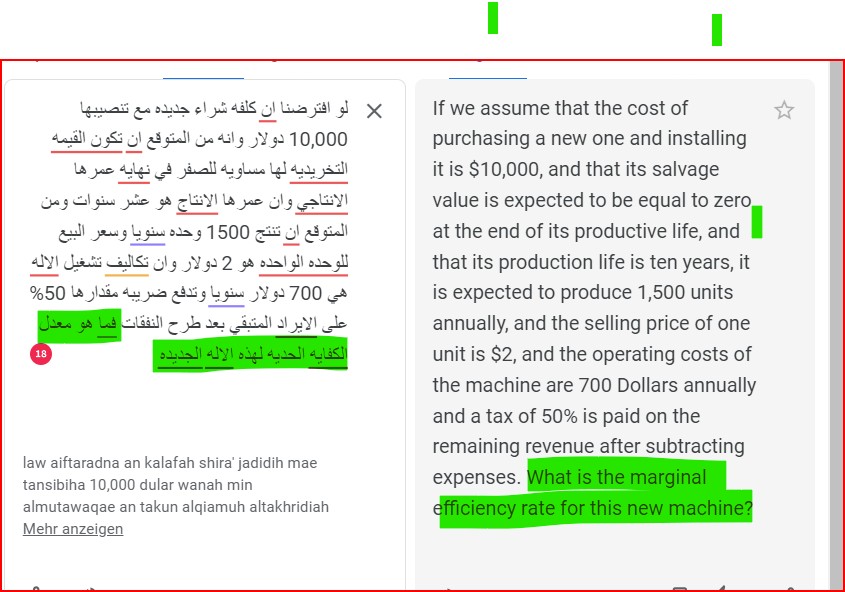
X لو الفترضنا ان كلفه شر اء جديده مع تنصيبها
law aiftaradna an kalafah shira' jadidih mae tansibiha 10,000 dular wanah min almutawaqae an takun alqiamuh altakhridiah Mehr anzeigen
If we assume that the cost of purchasing a new one and installing it is \( \$ 10,000 \), and that its salvage value is expected to be equal to zero at the end of its productive life, and that its production life is ten years, it is expected to produce 1,500 units annually, and the selling price of one unit is \( \$ 2 \), and the operating costs of the machine are 700 Dollars annually and a tax of \( 50 \% \) is paid on the remaining revenue after subtracting expenses. What is the marginal efficiency rate for this new machine?
Text erkannt:
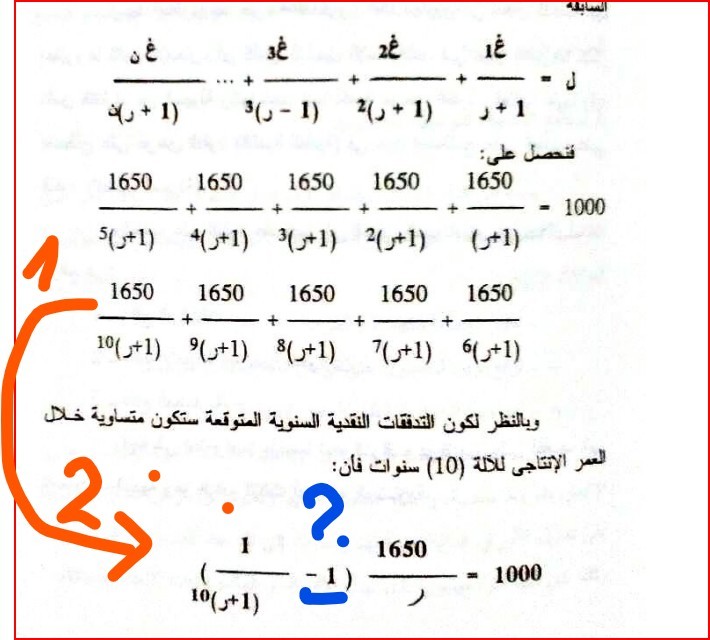
\( \begin{array}{l} \frac{1650}{{ }^{5}(\jmath+1)}+\frac{1650}{4(\jmath+1)}+\frac{1650}{{ }^{3}(\jmath+1)}+\frac{1650}{{ }^{2}(\jmath+1)}+\frac{1650}{(\jmath+1)}=1000 \\ \frac{1650}{{ }^{10}(\jmath+1)}+\frac{1650}{{ }^{9}(\jmath+1)}+\frac{1650}{{ }^{8}(\jmath+1)}+\frac{1650}{{ }^{7}(\jmath+1)}+\frac{1650}{{ }^{6}(\jmath+1)} \\ \end{array} \)
وبالنظر لكون اللنفقات النتية السنوية المتوتعة ستكون متساوية خلال
للعر الإتتاجى للكلة (10) سنوات نان:
\( \left(\frac{1}{10(\jmath+1)}-1\right) \frac{1650}{\jmath}=1000 \)