Aufgabe:
Bestimmung von phi
Text erkannt:
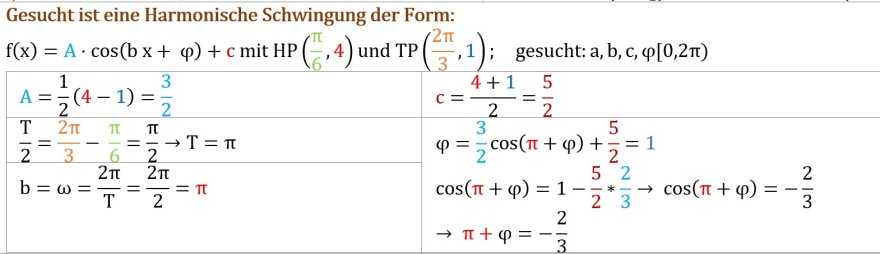
Gesucht ist eine Harmonische Schwingung der Form:
\( \begin{array}{l} \mathrm{f}(\mathrm{x})=\mathrm{A} \cdot \cos (\mathrm{bx}+\varphi)+\mathrm{c} \text { mit } \mathrm{HP}\left(\frac{\pi}{6}, 4\right) \text { und } \mathrm{TP}\left(\frac{2 \pi}{3}, 1\right) ; \text { gesucht: } \mathrm{a}, \mathrm{b}, \mathrm{c}, \varphi[0,2 \pi) \\ \begin{array}{|l|l} \mathrm{A}=\frac{1}{2}(4-1)=\frac{3}{2} & \mathrm{c}=\frac{4+1}{2}=\frac{5}{2} \\ \hline \frac{\mathrm{T}}{2}=\frac{2 \pi}{3}-\frac{\pi}{6}=\frac{\pi}{2} \rightarrow \mathrm{T}=\pi & \varphi=\frac{3}{2} \cos (\pi+\varphi)+\frac{5}{2}=1 \\ \mathrm{~b}=\omega=\frac{2 \pi}{\mathrm{T}}=\frac{2 \pi}{2}=\pi & \cos (\pi+\varphi)=1-\frac{5}{2} * \frac{2}{3} \rightarrow \cos (\pi+\varphi)=-\frac{2}{3} \\ & \rightarrow \pi+\varphi=-\frac{2}{3} \end{array} \end{array} \)
Problem/Ansatz:
Ich stehe gerade auf dem schlauch wie ich phi richtig berechnen muss