allgemeine Kreisgleichung:
$$ k: (x-c)^2+(y-d)^2=r^2 $$
Der Mittelpunkt hat die Koordinaten (c|d)
$$ x^2-8x+y^2+4y=30 $$
Mittels quadratischer Ergänzung ergibt sich:
$$ (x-4)^2-16+(y+2)^2-4=30 $$
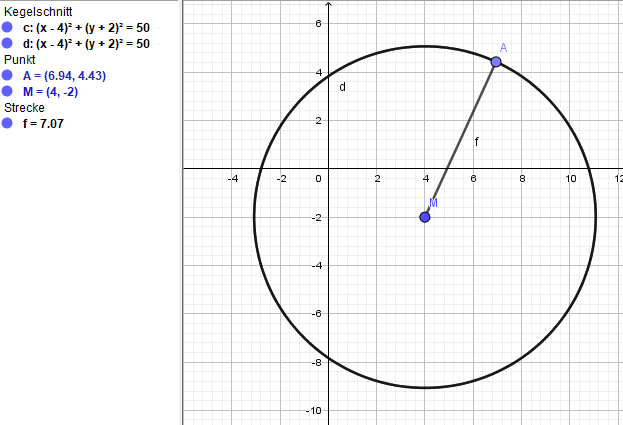
$$ (x-4)^2+(y+2)^2=50 $$
M (4|-2), Radius = 7,07
Gruß, Silvia