a) Der Graph von f ist rechtsgekrümmt und besitzt keinen Wendepunkt.
f(x)=\( \frac{1}{2} \) *(x-3)^2+4
b) Die Ableitungen f´ und f´´ haben nur negative Funktionswerte
f´´(x)=-x+5 → f´(x)=\( \int\limits_{}^{} \)(-x+5)*dx=0,5x^2+5x+C_1 → f(x) =\( \int\limits_{}^{} \)(0,5x^2+5x+C_1)*dx =\( \frac{1}{6} \)x^3+\( \frac{5}{2} \)x^2+C_1*x+C_2
Es sei nun C_1=2 und C_2=-\( \frac{1}{2} \)
f(x)=\( \frac{1}{6} \)x^3+\( \frac{5}{2} \)x^2+2*x-\( \frac{1}{2} \)
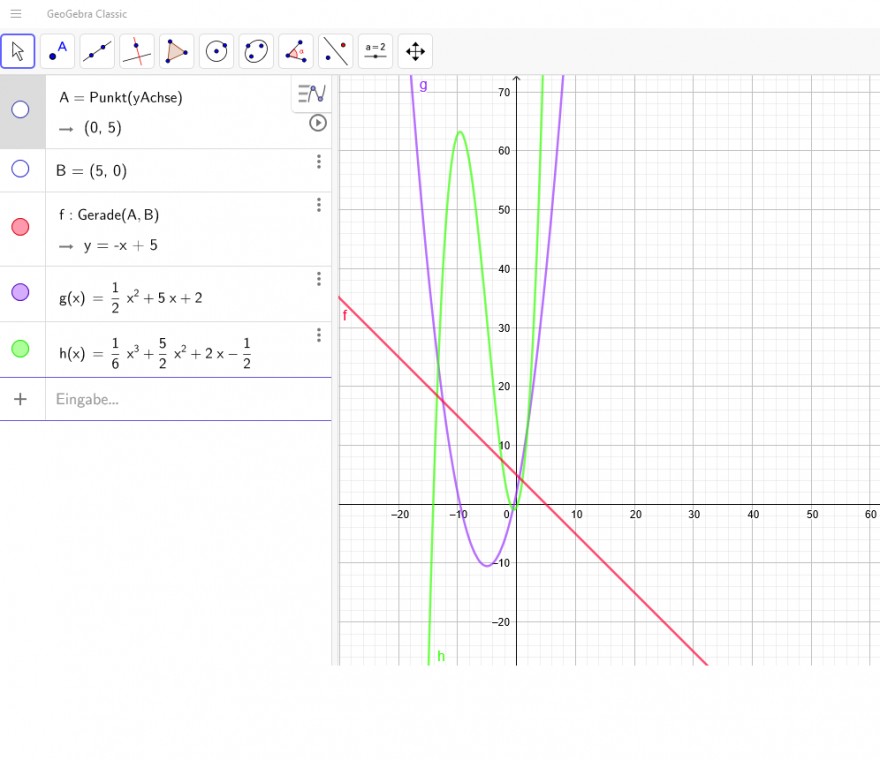
Text erkannt:
GeoGebra Classicic
\( \mathrm{A}= \) Punkt(yAchse)
\( \rightarrow(0,5) \)
\( B=(5,0) \)
\( f: \operatorname{Gerade}(A, B) \)
\( \rightarrow y=-x+5 \)
\( h(x)=\frac{1}{6} x^{3}+\frac{5}{2} x^{2}+2 x-\frac{1}{2} \)