Aufgabe: T26
Ich soll das Supremum und Infimum der Menge M2 bestimmen und falls vorhanden auch das Maximum und Minimum.
Problem/Ansatz: Ich verstehe nicht wie man auf die Umformung der Summenformel in den Lösungen kommt. 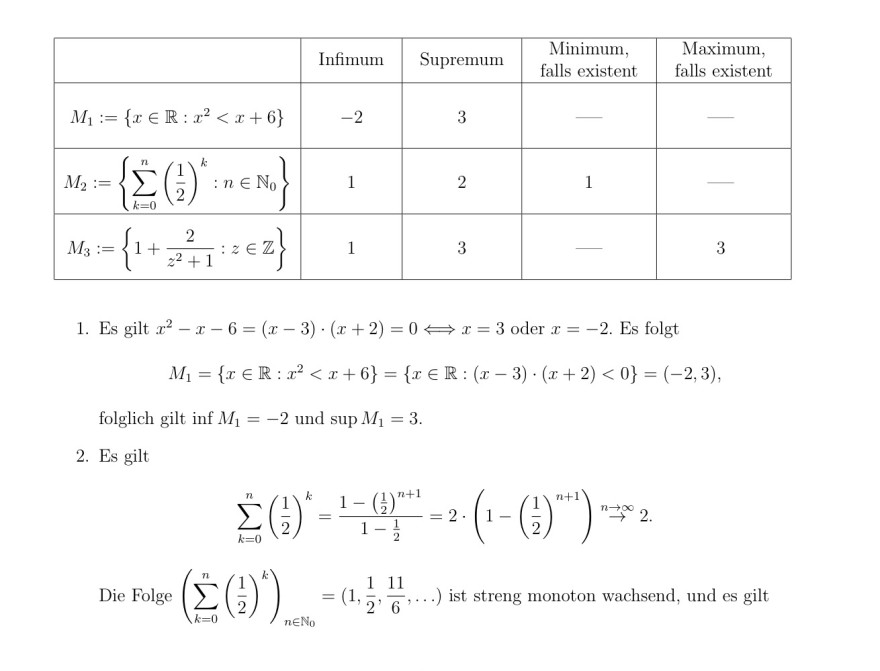
Text erkannt:
\(\begin{array}{|c|c|c|c|c|} \hline & \text{Infimum}&\text{ Supremum} & \text{Minimum, falls existent }& \text{Maximum, falls existent} \\ \hline M_{1}:=\left\{x \in \mathbb{R}: x^{2}<x+6\right\} & -2 & 3 & -& -\\ \hline M_{2}:=\left\{\sum \limits_{k=0}^{n}\left(\dfrac{1}{2}\right)^{k}: n \in \mathbb{N}_{0}\right\} & 1 & 2 & 1 & -\\ \hline M_{3}:=\left\{1+\dfrac{2}{z^{2}+1}: z \in \mathbb{Z}\right\} & 1 & 3 & - & 3\\ \hline \end{array}\)
1. Es gilt
\( x^{2}-x-6=(x-3) \cdot(x+2)=0 \Leftrightarrow x=3 \) oder \( x=-2 \).
Es folgt
\( M_{1}=\left\{x \in \mathbb{R}: x^{2}<x+6\right\}\\=\{x \in \mathbb{R}:(x-3) \cdot(x+2)<0\}\\=(-2,3), \)
folglich gilt
inf \( M_{1}=-2 \) und sup \( M_{1}=3 \).
2. Es gilt
\( \sum \limits_{k=0}^{n}\left(\frac{1}{2}\right)^{k}=\frac{1-\left(\frac{1}{2}\right)^{n+1}}{1-\frac{1}{2}}\\=2 \cdot\left(1-\left(\frac{1}{2}\right)^{n+1}\right)\stackrel{{n \to \infty}}{\longrightarrow}2 . \)
Die Folge
\( \left(\sum \limits_{k=0}^{n}\left(\frac{1}{2}\right)^{k}\right)_{n \in \mathbb{N}_{0}}=\left(1, \frac{1}{2}, \frac{11}{6}, \ldots\right) \)
ist streng monoton wachsend, und es gilt