Aufgabe:
Text erkannt:
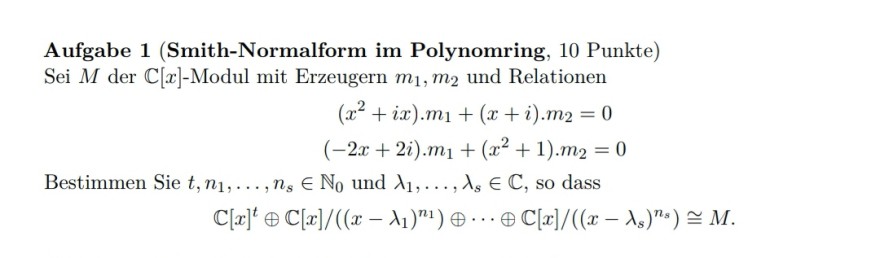
Aufgabe 1 (Smith-Normalform im Polynomring, 10 Punkte)
Sei \( M \) der \( \mathbb{C}[x] \)-Modul mit Erzeugern \( m_{1}, m_{2} \) und Relationen
\( \begin{array}{c} \left(x^{2}+i x\right) \cdot m_{1}+(x+i) \cdot m_{2}=0 \\ (-2 x+2 i) \cdot m_{1}+\left(x^{2}+1\right) \cdot m_{2}=0 \end{array} \)
Bestimmen Sie \( t, n_{1}, \ldots, n_{s} \in \mathbb{N}_{0} \) und \( \lambda_{1}, \ldots, \lambda_{s} \in \mathbb{C} \), so dass
\( \mathbb{C}[x]^{t} \oplus \mathbb{C}[x] /\left(\left(x-\lambda_{1}\right)^{n_{1}}\right) \oplus \cdots \oplus \mathbb{C}[x] /\left(\left(x-\lambda_{s}\right)^{n_{s}}\right) \cong M \)
Problem/Ansatz:
Bei der Aufgabe komme ich leider gar nicht weiter. Wie gehe ich da vor?
Danke schonmal im Voraus!