hey, wisst ihr vielleicht, wie man auf diese Darstellung "^3" für den Erwartungswert kommt?
Text erkannt:
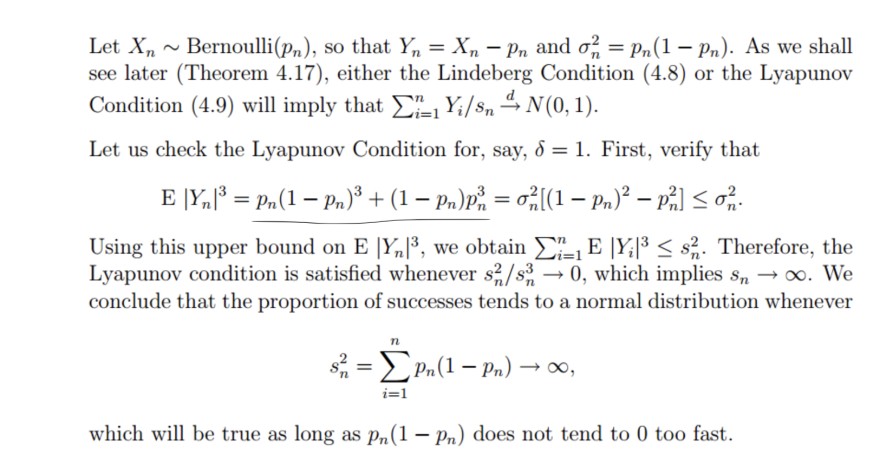
Let \( X_{n} \sim \operatorname{Bernoulli}\left(p_{n}\right) \), so that \( Y_{n}=X_{n}-p_{n} \) and \( \sigma_{n}^{2}=p_{n}\left(1-p_{n}\right) \). As we shall see later (Theorem 4.17), either the Lindeberg Condition (4.8) or the Lyapunov Condition (4.9) will imply that \( \sum \limits_{i=1}^{n} Y_{i} / s_{n} \stackrel{d}{\rightarrow} N(0,1) \)
Let us check the Lyapunov Condition for, say, \( \delta=1 \). First, verify that
\( \mathrm{E}\left|Y_{n}\right|^{3}=p_{n}\left(1-p_{n}\right)^{3}+\left(1-p_{n}\right) p_{n}^{3}=\sigma_{n}^{2}\left[\left(1-p_{n}\right)^{2}-p_{n}^{2}\right] \leq \sigma_{n}^{2} \)
Using this upper bound on \( \mathrm{E}\left|Y_{n}\right|^{3} \), we obtain \( \sum \limits_{i=1}^{n} \mathrm{E}\left|Y_{i}\right|^{3} \leq s_{n}^{2} \). Therefore, the Lyapunov condition is satisfied whenever \( s_{n}^{2} / s_{n}^{3} \rightarrow 0 \), which implies \( s_{n} \rightarrow \infty \). We conclude that the proportion of successes tends to a normal distribution whenever
\( s_{n}^{2}=\sum \limits_{i=1}^{n} p_{n}\left(1-p_{n}\right) \rightarrow \infty, \)
which will be true as long as \( p_{n}\left(1-p_{n}\right) \) does not tend to 0 too fast.
LG