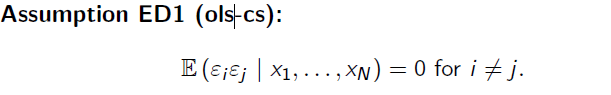
Text erkannt:
Assumption ED1 (ols-cs):
\( \mathbb{E}\left(\varepsilon_{i} \varepsilon_{j} \mid x_{1}, \ldots, x_{N}\right)=0 \text { for } i \neq j \)
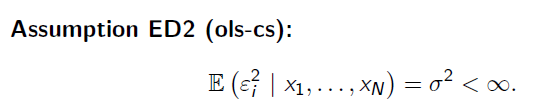
Text erkannt:
Assumption ED2 (ols-cs):
\( \mathbb{E}\left(\varepsilon_{i}^{2} \mid x_{1}, \ldots, x_{N}\right)=\sigma^{2}<\infty \)
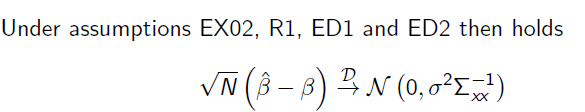
Text erkannt:
Under assumptions EX02, R1, ED1 and ED2 then holds
\( \sqrt{N}(\hat{\beta}-\beta) \stackrel{\mathcal{D}}{\rightarrow} \mathcal{N}\left(0, \sigma^{2} \Sigma_{x x}^{-1}\right) \)