Aufgabe:
Konstruieren Sie ein Polynom f, so dass
f(1) = 7, f(2) = 5 und f(3) = 6, grad(f)= 3 in Q[x],
Problem/Ansatz:
habe das Interpolationspolynom berechnet, dieses ist jedoch vom Grad 2. Das sieht man auch schon vor dem Kürzen (Siehe Bild)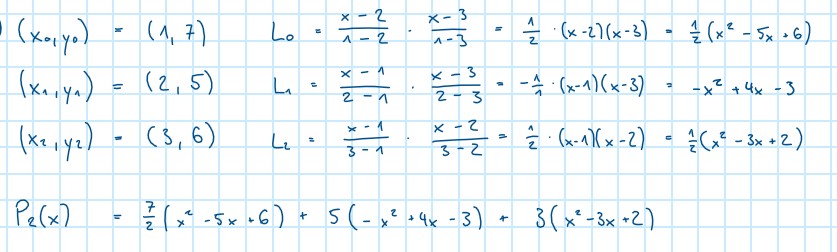
Text erkannt:
\( \begin{array}{l}\left(x_{0}, y_{0}\right)=(1,7) \quad L_{0}=\frac{x-2}{1-2} \cdot \frac{x-3}{1-3}=\frac{1}{2} \cdot(x-2)(x-3)=\frac{1}{2}\left(x^{2}-5 x+6\right) \\ \left(x_{1}, y_{1}\right)=(2,5) \quad L_{1}=\frac{x-1}{2-1} \cdot \frac{x-3}{2-3}=-\frac{1}{1} \cdot(x-1)(x-3)=-x^{2}+4 x-3 \\ \left(x_{2}, y_{2}\right)=(3,6) \quad L_{2}=\frac{x-1}{3-1} \cdot \frac{x-2}{3-2}=\frac{1}{2} \cdot(x-1)(x-2)=\frac{1}{2}\left(x^{2}-3 x+2\right) \\ P_{2}(x)=\frac{7}{2}\left(x^{2}-5 x+6\right)+5\left(-x^{2}+4 x-3\right)+3\left(x^{2}-3 x+2\right)\end{array} \)
Wie bringe ich es auf Grad 3 und was hat Q[x] mit dem ganzen zu tun?