accumulation point, limit inferior, divergent ect

Question

Aufgabe:

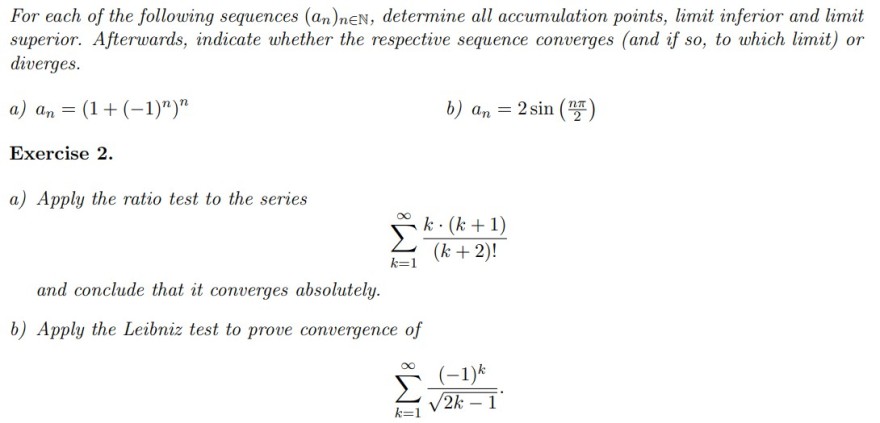
Text erkannt:

For each of the following sequences \( \left(a_{n}\right)_{n \in \mathbb{N}} \), determine all accumulation points, limit inferior and limit superior. Afterwards, indicate whether the respective sequence converges (and if so, to which limit) or diverges.
a) \( a_{n}=\left(1+(-1)^{n}\right)^{n} \)
b) \( a_{n}=2 \sin \left(\frac{n \pi}{2}\right) \)

Exercise 2.
a) Apply the ratio test to the series
\( \sum \limits_{k=1}^{\infty} \frac{k \cdot(k+1)}{(k+2) !} \)
and conclude that it converges absolutely.
b) Apply the Leibniz test to prove convergence of
\( \sum \limits_{k=1}^{\infty} \frac{(-1)^{k}}{\sqrt{2 k-1}} \)

Problem/Ansatz:

1a ) (-1) ^n führt ja zum periodischen wechsel   1 und -1   wenn ich das für 1^n einsetzte (n = 1 und 2) komme ich auf 2 und 0. heisst es die sequenz ist upperbounded von 2 und low bounded von 0 ? Accumulation point habe ich 4 und 0  indem ich für n 2k und 2k+1 einsetzte.  ist das falsch?

Comments

Du musst nochmal nachdenken, was Du geschrieben hast: Du sagst, eine obere Schranke sei 2 und ein Häufungspunkt sei 4 - wie soll das möglich sein?

Berechne doch mal die 6 ersten Folgenglieder.

Answer 1

Hallo

ja das ist falsch da du 2k nur innen, nicht außen eingesetzt hast, die 0 dagegen als HP und unteres limit ist richtig.

lul