Aufgabe:
Text erkannt:
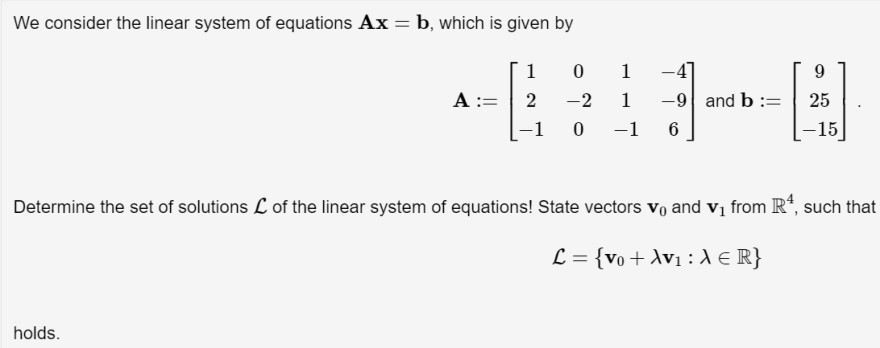
We consider the linear system of equations \( \mathbf{A x}=\mathbf{b} \), which is given by
\( \mathbf{A}:=\left[\begin{array}{cccc} 1 & 0 & 1 & -4 \\ 2 & -2 & 1 & -9 \\ -1 & 0 & -1 & 6 \end{array}\right] \text { and } \mathbf{b}:=\left[\begin{array}{c} 9 \\ 25 \\ -15 \end{array}\right] \text {. } \)
Determine the set of solutions \( \mathcal{L} \) of the linear system of equations! State vectors \( \mathbf{v}_{0} \) and \( \mathbf{v}_{1} \) from \( \mathbb{R}^{4} \), such that
\( \mathcal{L}=\left\{\mathbf{v}_{0}+\lambda \mathbf{v}_{1}: \lambda \in \mathbb{R}\right\} \)
holds.
Problem/Ansatz:
Gesucht ist v0 und v1 . Das obere Ax=b ist ja eine Koordinatenform und gesucht ist eine Parametereform. Heißt das ich kann mir eine der Koordinatenformen wie 1x1 + 1x3 -4x4 = 9 nehmen und umwandeln oder habe ich das falsch verstanden? Ich weiß nicht ganz wann man diese zero row umwandeln muss und wann nicht und warum.