Ich brauche eure Hilfe, ich komme garnicht bei der Aufgabe weiter, ich habe irgendwo einen Fehler gemacht aber finde den Fehler nicht, ich wollte so wie die Übungsleiterin die Aufgabe bearbeiten aber bei mir kommt etwas mit Wurzel ziehen aber ich darf das ja nicht mit dem TR machen

Text erkannt:
c)
\( p(x)=\frac{1}{2} x^{4}+x^{3} \)
Betrachte \( \tilde{p}(x)= \) ?
\( \begin{array}{l} \text { Mogl. NST } \quad P(x)=2 p(x)=x^{4}+2 x^{3}-3 x^{2}-4 x-4 \\ \text { Probe eraibt } \pm 4, \pm 2, \pm 1 \end{array} \)
Polynomadrision mit \( (x-2)(x+2)=x^{2}-4 \)
\( \begin{array}{l} \begin{array}{l} \left(x^{4}+2 x^{3}-3 x^{2}-8 x-4\right) \cdot\left(x^{2}-4\right)=x^{2}+2 x+1 \\ \left(-4 x^{4}\right) \end{array} \\ \frac{-\left(x^{4}-4 x^{2}\right)}{2 x^{3}+x^{2}} \\ \begin{aligned} & 2 x^{3}+x^{2}-8 x \\ - & \left(2 x^{3}\right. \end{aligned} \\ \frac{-\left(2 x^{3}-8 x\right.}{\left.x^{2}-8 x\right)} \\ \Rightarrow \bar{p}(x)=x^{2}+2 x+1=0 \\ \begin{array}{cc} -x^{2} & -4 \\ -\left(x^{2}\right. & -4) \\ 0 \end{array} \\ \text { Pg) } x_{1 / 2}=-1 \pm \sqrt{0}=-1 \\ \Rightarrow p(x)=\frac{1}{2}(x+1)^{2}(x-2)(x+2) \quad \begin{array}{l} \frac{\text { NSTV }}{2} / 1 \\ -2 / 1 \\ -1 / 2 \end{array} \end{array} \)

Text erkannt:
b) Bestimmen Sie alle Nullstellen (reell oder komplex) mit Vielfachheit und die vollständige Faktorisierung folgender Polynome:
i) \( p(x)=x^{4}-\frac{3}{2} x^{3}-\frac{1}{2} x^{2}+\frac{3}{2} x-\frac{1}{2} \),
ii) \( p(x)=x^{7}-2 x^{6}+4 x^{5}-6 x^{4}+3 x^{3} \),
iii) \( p(x)=-3 x^{4}+4 x^{3}+\frac{5}{3} x^{2}-4 x+\frac{4}{3} \).
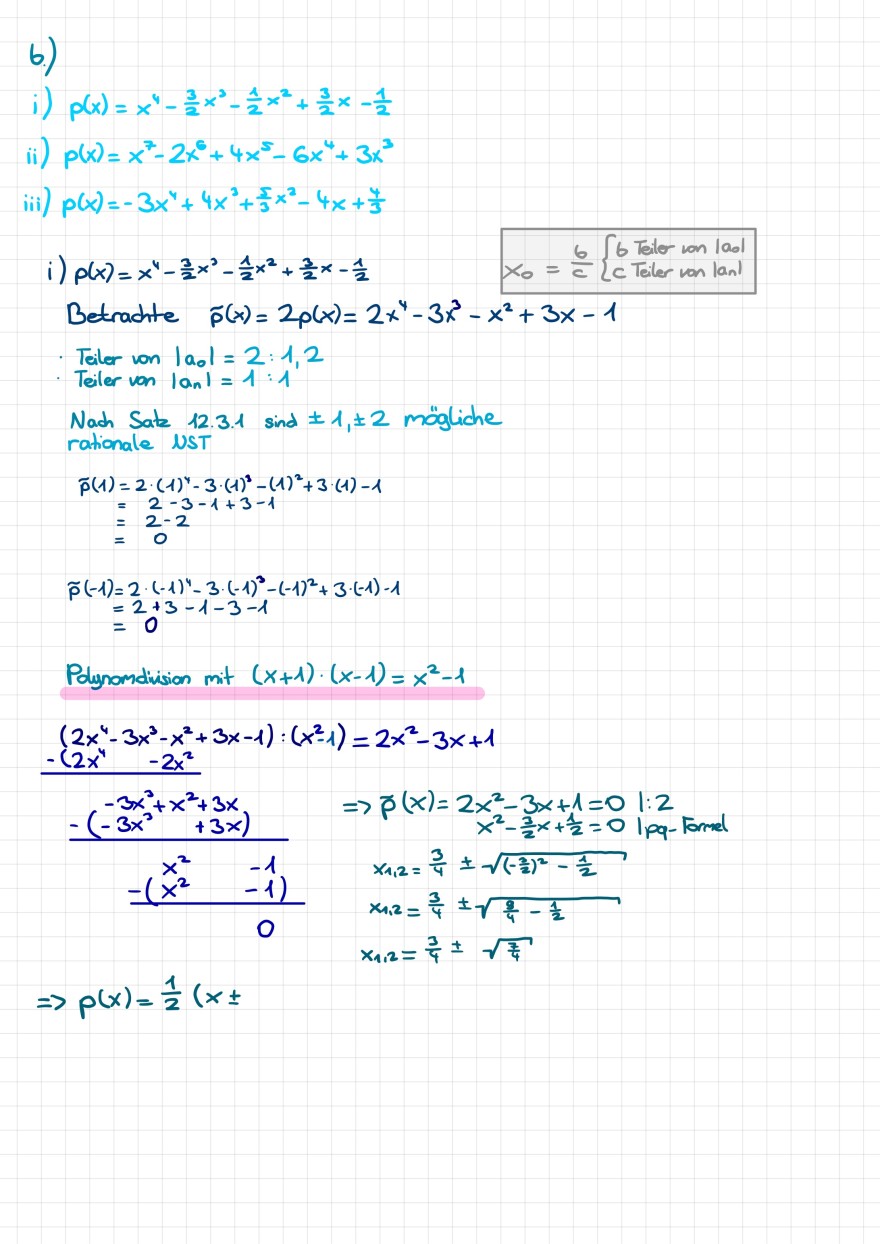
Text erkannt:
b.)
i) \( p(x)=x^{4}-\frac{3}{2} x^{3}-\frac{1}{2} x^{2}+\frac{3}{2} x-\frac{1}{2} \)
ii) \( p(x)=x^{7}-2 x^{6}+4 x^{5}-6 x^{4}+3 x^{3} \)
iii) \( p(x)=-3 x^{4}+4 x^{3}+\frac{5}{3} x^{2}-4 x+\frac{4}{3} \)
i) \( \rho(x)=x^{4}-\frac{3}{2} x^{3}-\frac{1}{2} x^{2}+\frac{3}{2} x-\frac{1}{2} \)
\( x_{0}=\frac{6}{c}\left\{\begin{array}{l}6 \text { Feilerion }|a o l| \\ c \text { Teiler van lan } \mid\end{array}\right. \)
Betrachte \( \tilde{p}(x)=2 p(x)=2 x^{4}-3 x^{3}-x^{2}+3 x-1 \)
Teiler von \( \left|a_{0}\right|=2: 1,2 \)
Teiler von \( \left|a_{n}\right|=1: 1 \)
Nach Satz 12.3.1 sind \( \pm 1, \pm 2 \) mögliche
rationale UST
\( \begin{aligned} \bar{p}(1) & =2 \cdot(1)^{4}-3 \cdot(1)^{2}-(1)^{2}+3 \cdot(1)-1 \\ & =2-3-1+3-1 \\ & =2-2-2 \\ & =0 \end{aligned} \)
\( \begin{aligned} \tilde{p}(-1) & =2 \cdot(-1)^{4}-3(-1)^{3}-(-1)^{2}+3 \cdot(-1)-1 \\ & =2+3-1-3-1 \\ & =0 \end{aligned} \)
Polynomdivision mit \( (x+1) \cdot(x-1)=x^{2}-1 \)
\( \begin{array}{l} \begin{array}{l} \left(2 x^{4}-3 x^{3}-x^{2}+3 x-1\right):\left(x^{2}-1\right)=2 x^{2}-3 x+1 \\ -\left(2 x^{4}-2 x^{2}\right. \end{array} \\ \frac{-\left(\begin{array}{c} -3 x^{3}+x^{2}+3 x \\ \left.-3 x^{3}+3 x\right) \end{array}\right.}{\frac{-\left(\begin{array}{ll} x^{2} & -1 \\ x^{2} & -1 \end{array}\right)}{0}} \\ \Rightarrow p(x)=2 x^{2}-3 x+1=0 \mid: 2 \\ x^{2}-\frac{3}{2} x+\frac{1}{2}=01 \mathrm{lpq} \text { - Forrel } \\ x_{1,2}=\frac{3}{4} \pm \sqrt{\left(-\frac{3}{2}\right)^{2}-\frac{1}{2}} \\ x_{1,2}=\frac{3}{4} \pm \sqrt{\frac{9}{4}-\frac{1}{2}} \\ x_{1,2}=\frac{3}{4} \pm \sqrt{\frac{7}{4}} \\ \Rightarrow p(x)=\frac{1}{2}(x \pm \end{array} \)