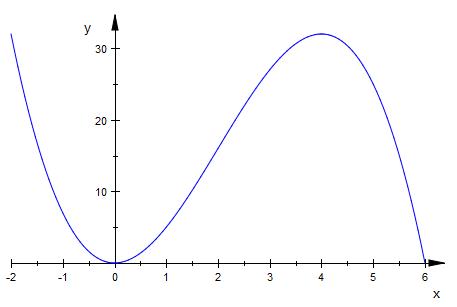
f ( x ) = -x3 + 6 * x2
f ´( x ) = -3*x^2 + 12 * x
Extremwerte
-3*x^2 + 12 * x = 0
x * ( -3 * x + 12 ) = 0
Ein Produkt ist dann 0 wenn mindestens einer der Faktoren 0 ist
x = 0
und
-3 * x + 12 = 0
x = 4
Monotonie > 0
-3*x^2 + 12 * x > 0
x * ( -3 * x + 12 ) > 0
1.Fall ( plus mal plus )
( x > 0 ) und ( -3 * x + 12 ) > 0
-3 * x + 12 > 0
12 > 3 * x
x < 4
Zusammen ( x > 0 ) und ( x < 4 ) => 0 < x < 4
Für 0 < x < 4 ist die Funktion steigend
2.Fall ( minus mal minus )
( x < 0 ) und ( -3 * x + 12 ) < 0
-3 * x + 12 < 0
12 < 3 * x
x > 4
Zusammen ( x < 0 ) und ( x > 4 ) : keine Schnittmenge
steigend : 0 < x < 4
fallend : x < 0 und x > 4