\(f(x) =\sqrt{-x^2-3x-2}\)
Nullstellen:
-x^2-3x-2 = 0
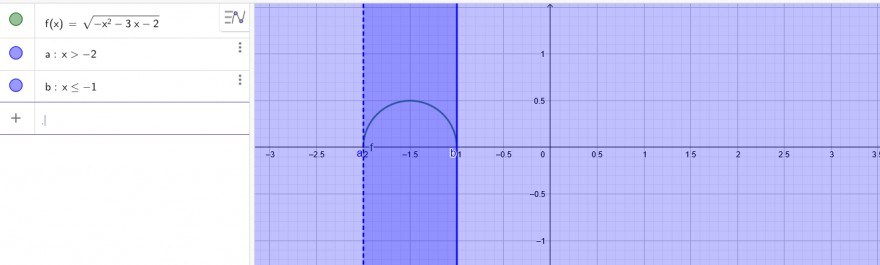
x₁=-2 ist nicht mehr im Bereich -2<x<-1/2 somit auch keine kleineren Werte als -2
x₂=-1 ist im Bereich -2<x<-1/2
x= - 0,9
\(f(-0,9) =\sqrt{-(-0,9)^2-3*(-0,9)-2}\)=\( \sqrt{-0,11} \) ist eine Lösung in ℂ
Somit ist der Definitionsbereich ( - 2 < x ≤ - 1]
(-x^2-3x-2)^1/2=-0,1|^2
-x^2-3x-2= 0,01
x^2+3x+2=-2, 01 Lösungen in ℂ Wertebereich y≥0 wie weit hängt vom Extremwert ab.
Text erkannt:
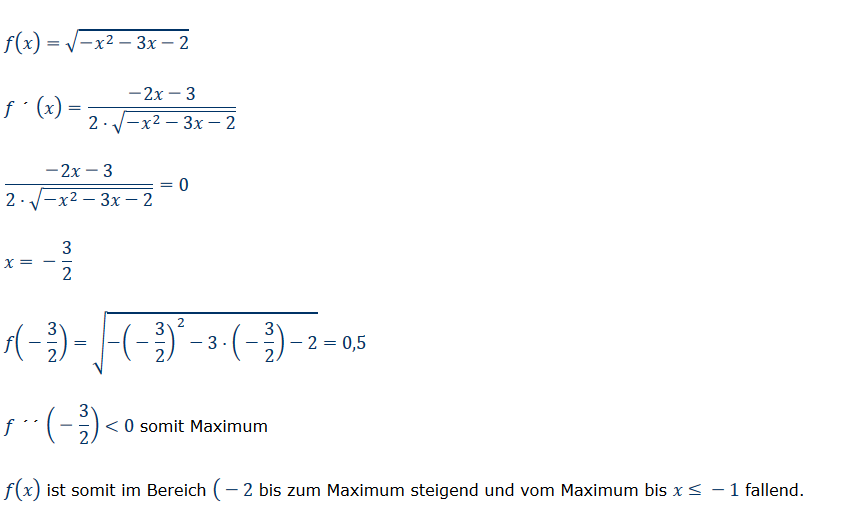
\( f(x)=\sqrt{-x^{2}-3 x-2} \)
\( f^{-}(x)=\frac{-2 x-3}{2 \cdot \sqrt{-x^{2}-3 x-2}} \)
\( \frac{-2 x-3}{2 \cdot \sqrt{-x^{2}-3 x-2}}=0 \)
\( x=-\frac{3}{2} \)
\( f\left(-\frac{3}{2}\right)=\sqrt{-\left(-\frac{3}{2}\right)^{2}-3 \cdot\left(-\frac{3}{2}\right)-2}=0,5 \)
\( f^{\cdots}\left(-\frac{3}{2}\right)<0 \) somit Maximum
\( f(x) \) ist somit im Bereich \( (-2 \) bis zum Maximum steigend und vom Maximum bis \( x \leq-1 \) fallend.