Bestimmen Sie sämtliche Nullstellen der Ableitungsfunktion.

Question

Aufgabe: Übungsaufgaben über Funktion (Extrema, Nullstellen, Intervalle, Monotonie, Grenzwerte & Folgen, Potenzen)

Problem/Ansatz:

Aufgabe 1.
Gegeben sei die Funktion f(x) = 1/4 x4 – x3 - 15/8 x2 -x-4, x ε R. Die Ableitungsfunktion hat bei x = - ½ eine Nullstelle.
Bestimmen Sie sämtliche Nullstellen der Ableitungsfunktion.
Geben Sie die Intervalle an, in denen f streng monoton wächst bzw. fällt.
Geben Sie die Extrema von f an.            

Aufgabe 2.
Gegeben sie die Folge (an)nεN , an = 2 -n -10, n ε N.
Geben Sie den Grenzwert der Folge an.
Bestimmen Sie den kleinsten Folgenindex derart, dass die ( absolute ) Abweichung aller Folgenterme ab diesem Index vom Grenzwert kleiner als 1/1000 ist.

Aufgabe 3.
Eine reelle Funktion werde in der Menge der reellen Zahlen definiert, indem jeder Zahl als Funktionswert die Differenz zugeordnet wird, die sich ergibt, in dem von der dritten Potenz der Zahl die Summe der Zahl und ihres Quadrates subtrahiert wird.
Stellen Sie den Funktionsterm auf.
Zeigen Sie, dass es genau eine Zahl gibt, für die der beschriebene Term den größten bzw. kleinsten Wert annimmt. Geben Sie diese Zahlen an.

Aufgabe 4.
Der für den Verkauf zuständige Manager eines Unternehmens prognostiziert, dass sich der Umsatz in den folgenden 12 Monaten ( August bis Juli des folgenden Jahres ) durch die Funktion f mit f(t) = t3 -21 t2 + 120t +200 (t in Monaten) beschreiben lässt.
Geben Sie die Zeiträume an, in denen nach dieser Prognose der Umsatz steigen bzw. fallen wird.
Berechnen Sie den nach dieser Prognose zu erwartenden größten bzw. kleinsten Umsatz. Zu welchen Zeitpunkten treten diese Umsätze ein?

Comments

Willst Du das wissen was im Titel steht, oder das was in der Aufgabe steht?

Answer 1

1. f ' (x) = x^3 - 3x^2 - 15x / 4 -1

hat eine Nullstelle bei -1/2 also durch (x+1/2) Polynomdivision machen,

das gibt x^2 - 3,5x - 2 und das =0 setzen gibt (pq-Formel)

x= -1/2  oder x=4

Von -∞ bis 4 fallend und danach steigend, also nur

ein Tiefpunkt bei T(4;-38)

Answer 2

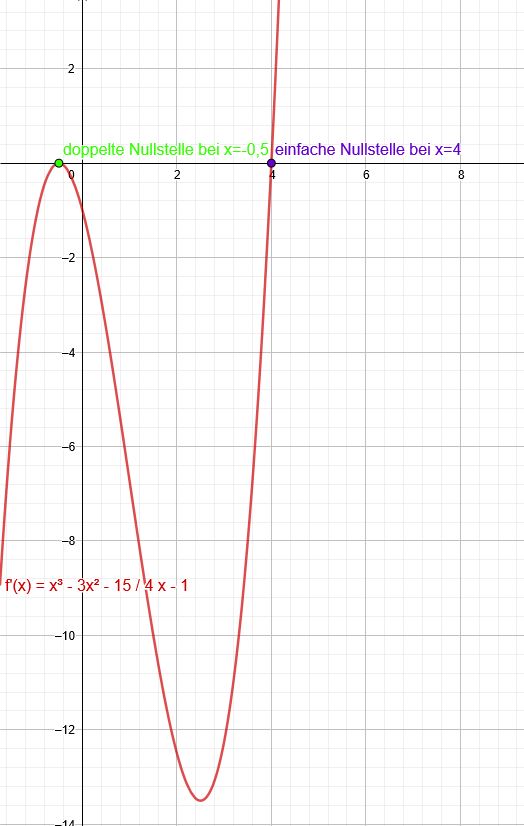
Nullstellen finden von:

\(f ' (x) = x^3 - 3x^2 - \frac{15}{4}x -1\)

\(f '' (x) = 3x^2 - 6x - \frac{15}{4}\)

\(3x^2 - 6x - \frac{15}{4}=0\)

\(x^2 - 2x - \frac{5}{4}=0\)

\(x^2 - 2x +\red{(\frac{2}{2})^2}=\frac{5}{4}+\red{(\frac{2}{2})^2}\)   \(\red{ quadratische Ergänzung}\)

\(x^2 - 2x +1=\frac{5}{4}+1=\frac{9}{4}\)   →2. Binom:

\((x-1)^2=\frac{9}{4}  |\sqrt{~~}\)

1.)

\(x-1=\frac{3}{2}  \)

\(x_1=1+\frac{3}{2} =2,5 \)    \(f ' (2,5) = (2,5)^3 - 3\cdot (2,5)^2 - \frac{15}{4}\cdot (2,5) -1=-13,5\) 

2.)

\(x-1=-\frac{3}{2}  \)

\(x_2=1-\frac{3}{2} =-0,5 \)  \(f ' (-0,5) = (-0,5)^3 - 3\cdot (-0,5)^2 - \frac{15}{4}\cdot (-0,5) -1=0\)

ist doppelte Nullstelle von \(f ' (x) \)