Text erkannt:
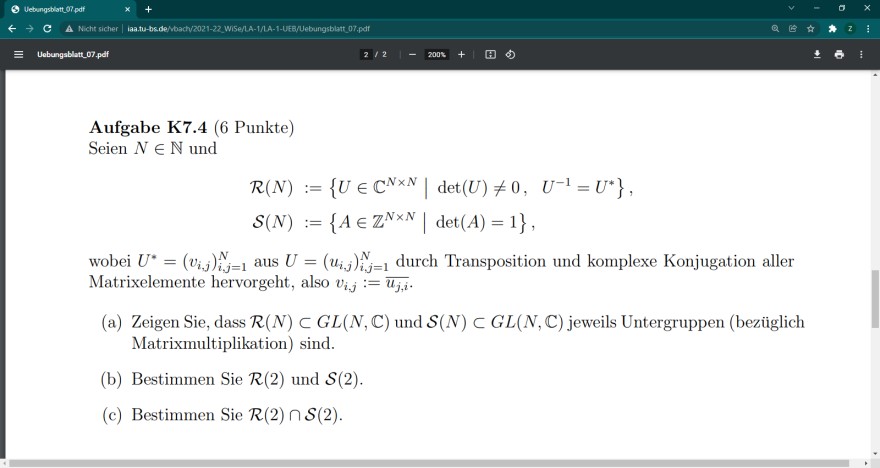
Aufgabe K7.4 (6 Punkte)
Seien \( N \in \mathbb{N} \) und
\( \begin{aligned} \mathcal{R}(N) &:=\left\{U \in \mathbb{C}^{N \times N} \mid \operatorname{det}(U) \neq 0, \quad U^{-1}=U^{*}\right\} \\ \mathcal{S}(N) &:=\left\{A \in \mathbb{Z}^{N \times N} \mid \operatorname{det}(A)=1\right\} \end{aligned} \)
wobei \( U^{*}=\left(v_{i, j}\right)_{i, j=1}^{N} \) aus \( U=\left(u_{i, j}\right)_{i, j=1}^{N} \) durch Transposition und komplexe Konjugation aller Matrixelemente hervorgeht, also \( v_{i, j}:=\overline{u_{j, i}} \).
(a) Zeigen Sie, dass \( \mathcal{R}(N) \subset G L(N, \mathbb{C}) \) und \( \mathcal{S}(N) \subset G L(N, \mathbb{C}) \) jeweils Untergruppen (bezüglich Matrixmultiplikation) sind.
(b) Bestimmen Sie \( \mathcal{R}(2) \) und \( \mathcal{S}(2) \).
(c) Bestimmen Sie \( \mathcal{R}(2) \cap \mathcal{S}(2) \).