Hm,
ich hab das ähnlich angegangen wie abakus
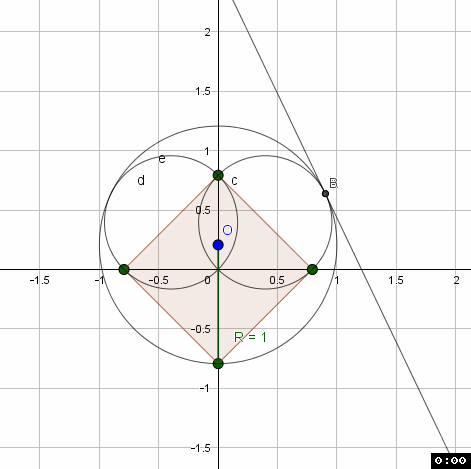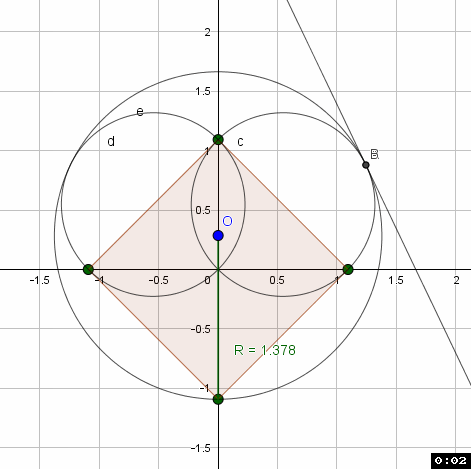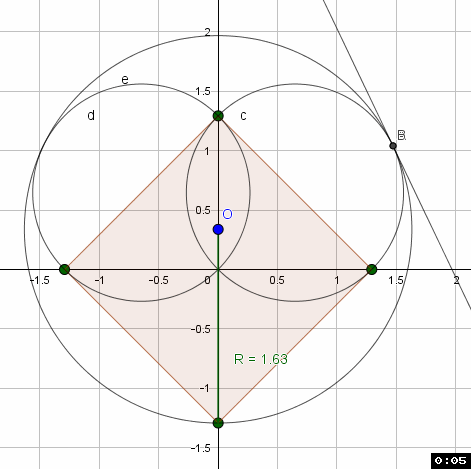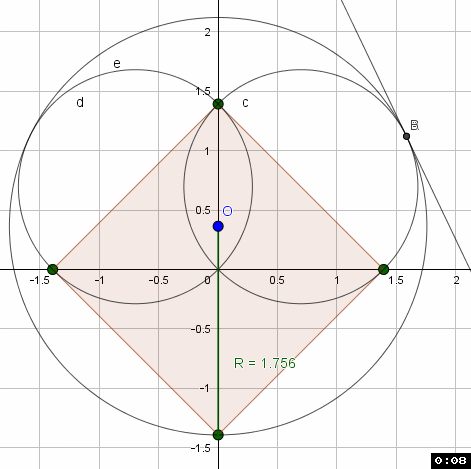
Quadrat_a={(0, -1), (1, 0), (0, 1), (-1, 0)}
K_R:(0,o)+ (o+a) (cos(t),sin(t))
k_r:(( (a) / 2, (a) / 2))+ a sqrt(2)/2 (cos(t),sin(t))
K_R = k_r
---- hässliche Rechnung (bekommt man vielleicht schöner hin, aber erstmal ein Ansatz)
\( R \, := \, a + \frac{1}{2} \cdot \frac{a \; \left(\sqrt{2} - 2 \right) \; \operatorname{cos} \left( 2 \; \operatorname{tan⁻^1} \left( \frac{1}{7} \; \left(\sqrt{2} - 3 \right) \right) \right) + a}{\operatorname{cos} \left( 2 \; \operatorname{tan⁻^1} \left( \frac{1}{7} \; \left(\sqrt{2} - 3 \right) \right) \right)} \)
\(r \, := \, a \; \frac{\sqrt{2}}{2}\)
\(r/R=\sqrt{2} \cdot \frac{\operatorname{cos} \left( 2 \; \operatorname{tan⁻^1} \left( \frac{1}{7} \; \left(\sqrt{2} - 3 \right) \right) \right)}{\sqrt{2} \; \operatorname{cos} \left( 2 \; \operatorname{tan⁻^1} \left( \frac{1}{7} \; \left(\sqrt{2} - 3 \right) \right) \right) + 1}=0.56066\)