matrix Gleichung lösen, ? wäre sehr dankbar

Question

Aufgabe:

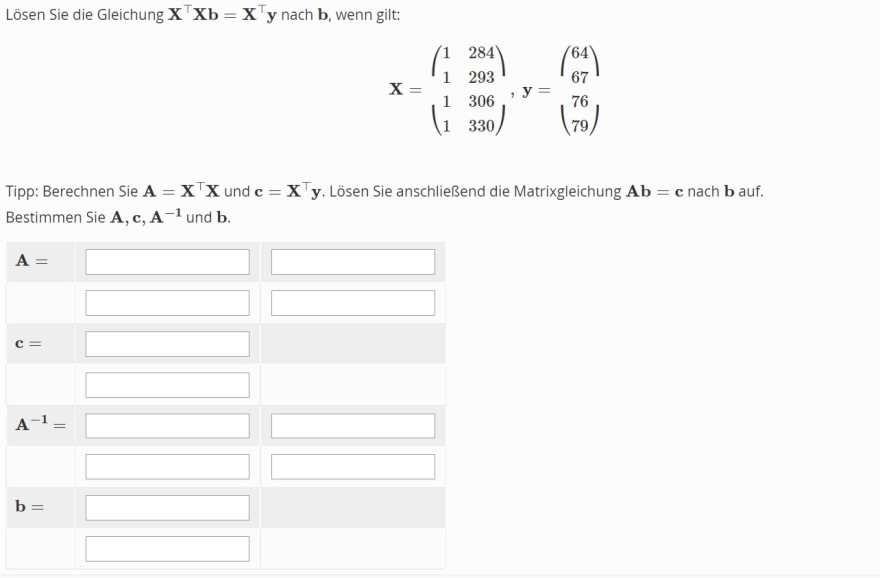
Text erkannt:

Lösen Sie die Gleichung \( \mathbf{X}^{\top} \mathbf{X} \mathbf{b}=\mathbf{X}^{\top} \mathbf{y} \) nach \( \mathbf{b} \), wenn gilt:
\( \left.\mathbf{X}=\left(\begin{array}{ll} 1 & 284 \\ 1 & 293 \end{array}\right),\left(\begin{array}{ll} 1 & 306 \\ 1 & 330 \end{array}\right), \mathbf{y}=\begin{array}{l} 64 \\ 67 \end{array}\right) \)
Tipp: Berechnen Sie \( \mathbf{A}=\mathbf{X}^{\top} \mathbf{X} \) und \( \mathbf{c}=\mathbf{X}^{\top} \mathbf{y} \). Lösen Sie anschließend die Matrixgleichung \( \mathbf{A} \mathbf{b}=\mathbf{c} \) nach \( \mathbf{b} \) auf. Bestimmen Sie \( \mathbf{A}, \mathbf{c}, \mathbf{A}^{-1} \) und \( \mathbf{b} \).
\( \mathbf{A}= \)
\( \mathbf{c}= \)
\( \mathbf{A}^{-1}= \)
\( \mathbf{b}= \)

Problem/Ansatz:

hallo, 3 aufgaben habe ich schon gelöst, da komme ich aber nicht weiter. Könnte mir jemand helfen?

Answer 1

Hallo Jenny,

es ist:$$A= X^T\cdot X = \begin{pmatrix}4& 1213\\ 1213& 369041\end{pmatrix}\\ c = X^T \cdot y = \begin{pmatrix}286\\ 87133\end{pmatrix} \\ A^{-1} = \frac{1}{4795}\begin{pmatrix}369041& -1213\\ -1213& 4\end{pmatrix} \\ b = \frac{1}{4795}\begin{pmatrix}-146603\\ 1614\end{pmatrix}$$Gruß Werner

Comments

Danke sehr :)

---

das stimmt leider nicht :( ist nicht korrekt

---

das stimmt leider nicht :( ist nicht korrekt

woraus schließt Du das?

https://www.desmos.com/calculator/flw3tl8bbz

das sieht aber ziemlich richtig aus. Ich habe das ganze nochmal mit Excel überprüft. Die Ergebnisse stimmen mit meinen exakt überein.

Wenn man den Achsenabschnitt \(b\) (alias \(b_1\) und die Steigung \(m\) (alias \(b_2\)) der Geraden näherungsweise angibt, kommt man zu$$b=−30,5741397289\\m=0,336600625652$$Vielleicht liegt's am Dezimaltrenner (Komma oder Punkt) oder geforderten Anzahl der Nachkommastellen?

---

da ich Vorbereitung für Klausur gemacht habe, Selbsttest gelöst und da ich genauso die Ergebnisse eingegeben und leider keine Punkte erhalten. es kann auch Systemfehler sein. :(

werde nächste Woche Prof. fragen

danke sehr