Aufgabe:Zeige, dass f diff'bar ist und berechne die Jacobsmatrix
Problem/Ansatz:
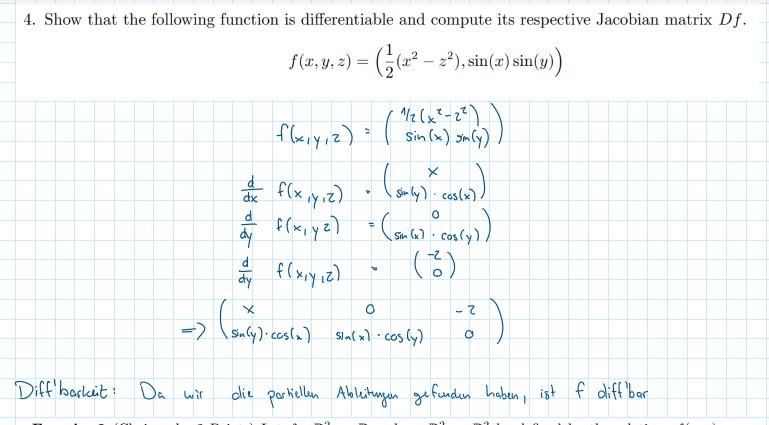
Text erkannt:
4. Show that the following function is differentiable and compute its respective Jacobian matrix \( D f \).
\( \begin{array}{l} f(x, y, z)=\left(\frac{1}{2}\left(x^{2}-z^{2}\right), \sin (x) \sin (y)\right) \\ f(x, y, z)=\left(\begin{array}{c} 1 / 2\left(x^{2}-z^{2}\right) \\ \sin (x) \sin (y) \end{array}\right) \\ \frac{d}{d x} f(x, y, z) \cdot\left(\begin{array}{c} x \\ \sin (y) \cdot \cos (x) \end{array}\right) \\ \frac{d}{d y} f(x, y z)=\left(\begin{array}{c} 0 \\ \sin (x) \cdot \cos (y) \end{array}\right) \\ \frac{d}{d y} f(x, y, z)=\left(\begin{array}{c} -z \\ 0 \end{array}\right) \\ \Rightarrow\left(\begin{array}{ccc} x & 0 & -2 \\ \sin (y) \cdot \cos (x) & \sin (x) \cdot \cos (y) & 0 \end{array}\right) \\ \end{array} \)
Diff'barkit: Da wir die partielken Ablitumgan gefunden haben, ist \( f \) diff'bar
habe ich die Aufgabe korrekt gelöst? Bei der diff'barkeit wäre das wohl vermutlich nicht ausreichend, was müsste ich noch hinzufügen?