Aufgabe:
Darstellungsmatrix von B nach C
Problem/Ansatz:
Mein Ansatz war es die Darstellungsmatrix als Linearkombination der Basisvektoren von B darzustellen, jedoch hat dies nicht geklappt wie gewollt. Um das ganze als Matrix darzustellen habe ich für die B die Matrix: {{-1,1,1,-1},{1,-2,-3,1},{-1,1,2,-1},{1,-1,-2,0}} und C als Matrix: {{-1,1,1,-1},{1,-2,-3,1},{-1,1,2,-1},{1,-1,-2,0}} erstellt. Dieser Ansatz scheint jedoch falsch zu sein, da ich auf beim Endergebnis nicht auf die gewünschte 3x4 Matrix komme.
Ich wäre dankbar über jede Erklärung und Hilfestellung.
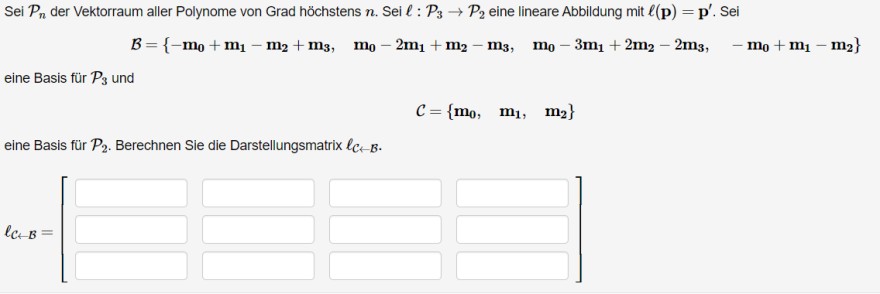
Text erkannt:
Sei \( \mathcal{P}_{n} \) der Vektorraum aller Polynome von Grad höchstens \( n \). Sei \( \ell: \mathcal{P}_{3} \rightarrow \mathcal{P}_{2} \) eine lineare Abbildung mit \( \ell(\mathbf{p})=\mathbf{p}^{\prime} \). Sei
\( \mathcal{B}=\left\{-\mathbf{m}_{0}+\mathbf{m}_{1}-\mathbf{m}_{2}+\mathbf{m}_{3}, \quad \mathbf{m}_{0}-2 \mathbf{m}_{1}+\mathbf{m}_{2}-\mathbf{m}_{3}, \quad \mathbf{m}_{0}-3 \mathbf{m}_{1}+2 \mathbf{m}_{2}-2 \mathbf{m}_{3}, \quad-\mathbf{m}_{0}+\mathbf{m}_{1}-\mathbf{m}_{2}\right\} \)
eine Basis für \( \mathcal{P}_{3} \) und
\( \mathcal{C}=\left\{\mathbf{m}_{0}, \quad \mathbf{m}_{\mathbf{1}}, \quad \mathbf{m}_{\mathbf{2}}\right\} \)
eine Basis für \( \mathcal{P}_{2} \). Berechnen Sie die Darstellungsmatrix \( \ell_{\mathcal{C} \leftarrow \mathcal{B}} \).
\( \ell_{\mathcal{C} \leftarrow \mathcal{B}}=\left[\begin{array}{l|l|lll} & & & & \\ & & & & \\ & & & & \\ & & & & \\ & & & & \end{array}\right] \)