Komplexe Matrix invertieren

Question

Text erkannt:

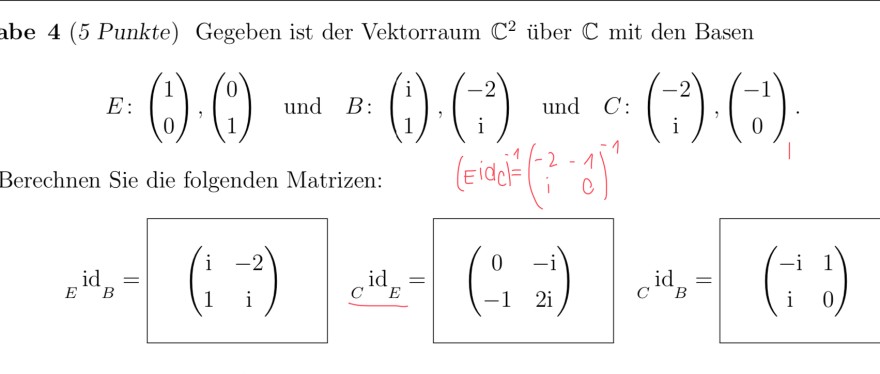
abe 4 ( 5 Punkte) Gegeben ist der Vektorraum \( \mathbb{C}^{2} \) über \( \mathbb{C} \) mit den Basen
\( E:\left(\begin{array}{l} 1 \\ 0 \end{array}\right),\left(\begin{array}{l} 0 \\ 1 \end{array}\right) \text { und } B:\left(\begin{array}{l} \mathrm{i} \\ 1 \end{array}\right),\left(\begin{array}{c} -2 \\ \mathrm{i} \end{array}\right) \text { und } C:\left(\begin{array}{c} -2 \\ \mathrm{i} \end{array}\right),\left(\begin{array}{c} -1 \\ 0 \end{array}\right) \text {. } \)

Berechnen Sie die folgenden Matrizen:
\( { }_{E} \mathrm{id}_{B}=\left(\begin{array}{cc} \mathrm{i} & -2 \\ 1 & \mathrm{i} \end{array}\right) \quad{ }_{C} \mathrm{id}_{E}=\quad\left(\begin{array}{cc} 0 & -\mathrm{i} \\ -1 & 2 \mathrm{i} \end{array}\right) \quad{ }_{C} \mathrm{id}_{B}=\quad\left(\begin{array}{cc} -\mathrm{i} & 1 \\ \mathrm{i} & 0 \end{array}\right) \)

Hallo, ich möchte gerne die inverse einer komplexen Matrix angeben. Das Ergebnis ist gegeben, allerdings ist mir das Vorgehen unklar. Könnte mir das bitte jemand erklären?

LG

Answer 1

Die berechnet sich genauso wie im Reellen, da gibt es eine Formel:

https://www.massmatics.de/merkzettel/#!305:Inverse_von_2x2-Matrizen

Es ist nützlich, sich das zu merken. Dass die Formel stimmt, kann man mit der Probe überprüfen.

Wenn es man selbst herleiten will, das geht genauso wie im Reellen (Zeilenstufenform usw.).