Integral von sin x cos x e^{sin^2 x} mit Substitution

Question

Berechnen Sie das bestimmte Integral in a) mit Hilfe einer geeigneten Substitution und das unbestimmte Integral in b) mit partieller Integration.

a) \( \int \limits_{0}^{\frac{\pi}{4}} \sin x \cos x e^{\sin ^{2} x} \mathrm{dx} \)

b) \( \int x^{2} \cdot e^{-2 x} \mathrm{dx} \)

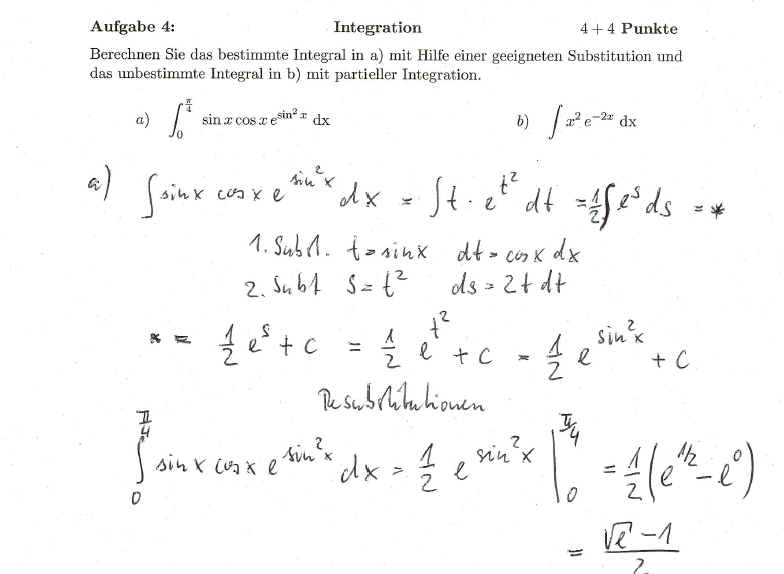
Kann mir jemand die Rechenschritte hier erklären?

Integral von sin x cos x e^{sin^2 x} mit Substitution

Answer 1

Hier steht doch praktisch die innere Ableitung als Faktor vor e^u

u = sin^2 x

du / dx = 2*sin x * cos x

0.5 du = sinx cos x dx

f(u) = e^{u}

f' (u) = e ^{u}

Gesuchtes Integral =0.5 ∫ e^u du = 0.5 e^u + C

= 0.5 e^{sin^2 x} + C

Comments

Am Schluss noch die Grenzen einsetzen.

sin(π/4) = 1/√2

sin(0) = 0