fa(x) = 4/3 * x^3 - a * x^2
f ´( x ) = 4 * x^2 - 2a * x
f ´´ ( x ) = 8 * x - 2a
Stellen mit waagerechter Tangente
4x^2 - 2ax = 0
x * ( 4x - 2a ) = 0
x = 0
und
4x - 2a = 0
x = 1/2 * a
Min oder max
f ´´ ( 0 ) = - 2a
f ´´ ( 1/2 a ) = 8 * 1/2 * a - 2a
f ´´ ( 1/2 a ) = 4 * a - 2a
f ´´ ( 1/2 a ) = 2a
Ob eine Extremstelle Min oder Max ist
hängt von a ab.
Für a > 0 ist
f ´´ ( 0 ) = Hochpunkt
f ´´ ( 1/2 a ) = Tiefpunkt
Ich rechne einmal für diesen Fall
x = 1/2 * a
y = - a^3 / 12
x = 1/2 * a => a = 2*x
Einsetzen
y = -(2x)^3 / 12
ort ( x ) = -8x^3 / 12
ort ( x ) = - 2/3 * x^3
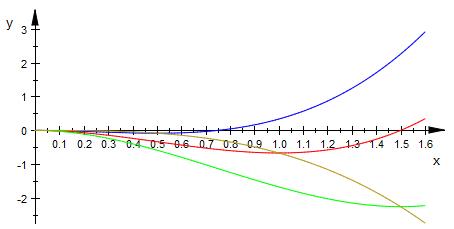
blau, rot, grün sind die Graphen für
a=1, 2, 3
oker ist die Ortskurve welche alle Tiefpunkte
miteinander verbindet.