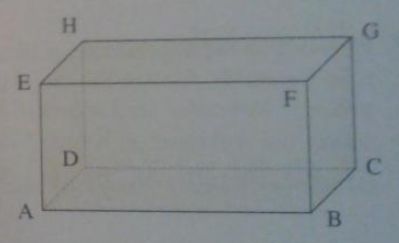
Prüfen Sie anhand des abgebildeten Quaders, ob die gegebenen drei Vektoren komplanar sind.
a) \( \overrightarrow{\mathrm{AB}}, \overrightarrow{\mathrm{AE}}, \overrightarrow{\mathrm{AF}} \)
b) \( \overrightarrow{\mathrm{AB}}, \overrightarrow{\mathrm{CG}}, \overrightarrow{\mathrm{EA}} \)
c) \( \overrightarrow{\mathrm{AE}}, \overrightarrow{\mathrm{DC}}, \overrightarrow{\mathrm{FG}} \)
d) \( \overrightarrow{\mathrm{HE}}, \overrightarrow{\mathrm{BC}}, \overrightarrow{\mathrm{AG}} \)
e) \( \overrightarrow{\mathrm{AC}}, \overrightarrow{\mathrm{FG}}, \overrightarrow{\mathrm{HD}} \)
Ansatz:
(b) komplanar
(d) komplanar
Ich dachte bisher, komplanare Vektoren befänden sich in einer Ebene. Das sind die Vektoren in (b) und (d) doch gar nicht! Oder gibt es noch eine andere Form von Komplanarität, die ich nicht kenne?