Nicht lineares gleichungssystem mit newton lösen

Question

Text erkannt:

Aufgabe 2 [Nichtlineares Gleichungssystem, Newton-Verfahren] Die Parameter \( a, b, c \) der Funktion \( f(t)=a+b \sin (c+t) \) sollen aus gegebenen Werten \( f\left(t_{i}\right)=y_{i}, i=1,2,3 \) bestimmt werden.
(a) Stellen Sie ein entsprechendes nichtlineares Gleichungssystem \( F(a, b, c)=y \in \mathbb{R}^{3} \) auf.
(b) Geben Sie die Newton-Iteration zur Lösung des Gleichungssystems an.

Kann mir jemand bei der Aufgabe helfen, Ich verstehe den Ansatz nicht...???

Answer 1

Kennst Du denn das Newton Verfahren?

Comments

Ja so semi, also hatten es zuletzt in der VL....

---

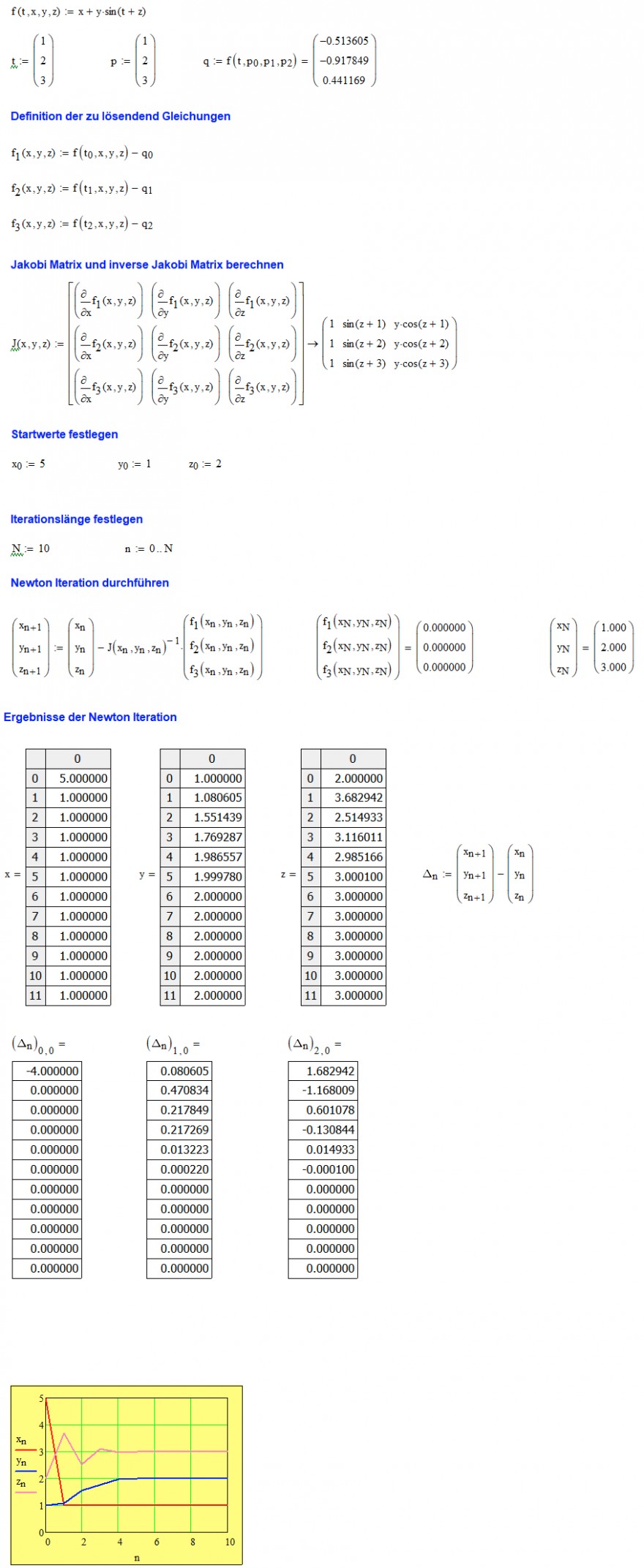
Die Gleichungen lauten

$$  f(t_i,a,b,c) - y_i = 0 $$ Das sind drei Gleichungen für \( i=1,2,3 \)

Das Newtonverfahren sieht so aus

$$ \begin{pmatrix} a\\b\\c \end{pmatrix}_{n+1} = \begin{pmatrix} a\\b\\c \end{pmatrix}_{n} - J(a,b,c)^{-1} \left( f(t_i, a_n ,b_n, c_n) - y_i \right) $$

\( J \) ist die Jakobi Matrix bzgl.\( a, b, c\)

Hier noch ein numerisches Beispiel