Schwierigkeiten beim beweisen der Rechenregeln

Question

Hallo, ich soll folgende Rechenregeln beweisen.

Körperaxiome:

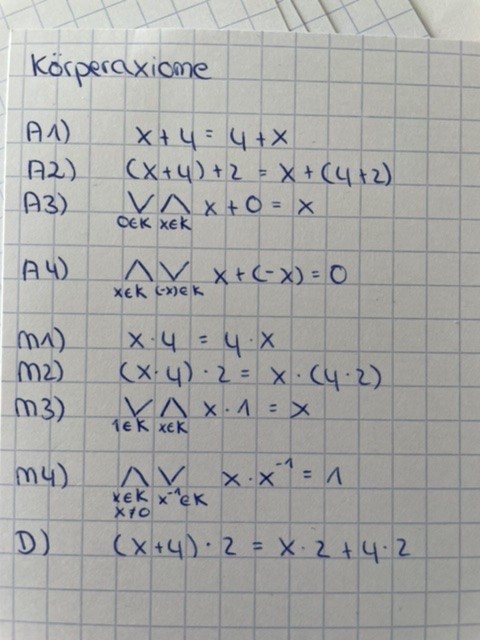
Aufgabe 3: \( \mathrm{K} \) sei ein Körper. Zeigen Sie:

(a) Für alle \( \mathrm{x} \in \mathrm{K} \) gilt: \( \mathrm{x} \cdot 0=0 \).
(b) Für alle \( x \in K \) gilt; \( -(-x)=x \).
(c) Für alle \( x, y \in K \) gilt: \( (-x) \cdot y=-(x \cdot y) \).
(d) Für alle \( x, y \in K \) gilt \( :(-x) \cdot(-y)=x \cdot y \),
(e) Für alle \( x, y \in K \) gilt: \( x \cdot y=0 \Rightarrow x=0 \vee y=0 \).
(f) Für alle \( x, y \in K \) gilt: \( (x+y)^{2}=x^{2}+2 \cdot x \cdot y+y^{2} \).

Problem/Ansatz:

Leider fällt es mir unglaublich schwer, diese Regeln zu beweisen, eventuell könnte ich es nachvollziehen, wenn ich mal gesehen habe wie es funktioniert.

Wäre über jede Hilfe sehr dankbar.

Liebe Grüße

Comments

Das Wesen aller Axiome ist, dass sie als gültig vorausgesetzt werden, also nie bewiesen werden. Wenn man aber die Axiome auf ein anderes System von Axiomen zurückführt, dann werden es Sätze und sind in dem anderen Axiomensystem beweisbar. Dazu müsste man aber das andere Axiomensystem kennen.

---

Hört sich so an, als hättest du die Aufgabe überhaupt nicht verstanden.

---

Warum weist du nur auf mögliche Ungereimtheiten in der Antwort hin und antwortest nicht selbst?

---

wäre denn eventuell folgendes eine richtige Lösung für b)?

Oder müsste ich noch etwas beweisen?

Oder ist das sogar komplett falsch?

---

Solche Quantoren habe ich das letzte mal vor 30 Jahren in der Schule gesehen.

Übrigens sieht dein \(y\) aus wie eine 4 und dein \(z\) wie eine 2. Hat mich anfangs ordentlich verwirrt.

---

Ich finde es auch schade, dass sie heute nicht mehr so populär sind.

Answer 1

(a)

\(\begin{aligned} &  & x\cdot0 & =x\cdot0\\ & \stackrel{\text{A3}}{\implies} & x\cdot(0+0) & =x\cdot0\\ & \stackrel{\text{D}}{\implies} & \left(x\cdot0\right)+\left(x\cdot0\right) & =x\cdot0 &  & |\,+\left(-\left(x\cdot 0\right)\right)\\ & \stackrel{}{\implies} & \left(\left(x\cdot0\right)+\left(x\cdot0\right)\right)+\left(-\left(x\cdot0\right)\right) & =\left(x\cdot0\right)+\left(-\left(x\cdot0\right)\right)\\ & \stackrel{\text{A2}}{\implies} & \left(x\cdot0\right)+\left(\left(x\cdot0\right)+\left(-\left(x\cdot0\right)\right)\right) & =\left(x\cdot0\right)+\left(-\left(x\cdot0\right)\right)\\ & \stackrel{\text{A4}}{\implies} & \left(x\cdot0\right)+0 & =0\\ & \stackrel{\text{A3}}{\implies} & x\cdot0 & =0 \end{aligned}\)

(b)

\(\begin{aligned} & \text{A4} & x+\left(-x\right) & =0 &  & |+\left(-\left(-x\right)\right)\\ & \implies & \left(x+\left(-x\right)\right)+\left(-\left(-x\right)\right) & =0+\left(-\left(-x\right)\right)\\ & \stackrel{\text{A1}}{\implies} & \left(x+\left(-x\right)\right)+\left(-\left(-x\right)\right) & =\left(-\left(-x\right)\right)+0\\ & \stackrel{\text{A3}}{\implies} & \left(x+\left(-x\right)\right)+\left(-\left(-x\right)\right) & =-\left(-x\right)\\ & \stackrel{\text{A2}}{\implies} & x+\left(\left(-x\right)+\left(-\left(-x\right)\right)\right) & =-\left(-x\right)\\ & \stackrel{\text{A4}}{\implies} & x+0 & =-\left(-x\right)\\ & \stackrel{\text{A3}}{\implies} & x & =-\left(-x\right) \end{aligned}\)