Größtmögliches Intervall angeben auf dem die Funktion streng monoton fallend ist

Question

Aufgabe:

Geben Sie das größtmögliche Intervall an, auf dem die Funktion \( f \) mit

\( f(x)=2 x^{3}-150 x+5 \)

streng monoton fallend ist.

Problem/Ansatz:

Hallöle,

Kann mir wer eine Lösung mit Erklärung zeigen, ich komme auf die Falsche lösung, ich habe die werte der nullten herausgenommen und in der erste ableitung eingestezt aber komme nicht auf die Richtige Lösung

Answer 1

Etwas ausführlicher.

f ( x ) = 2 * x^3  - 150 * x + 5
1.Ableitung = Steigung
f ´( x ) = 6 * x^2 - 150
Festellen wo die Steigung null ist
6 * x^2 - 150 = 0
6 * x^2 = 150
x^2 = 25

x = +5
und
x = -5

( Jetzt ohne Nachweis. Die Stellen
sind Extremstellen. )

Es gibt jetzt -zig Möglichkeiten wie man weiter
rechnet.
Wir schauen uns an wie die Steigung in einem
Punkt zwischen x = - 5 und x = 5 ist.
Für x = 0 gilt
f ´( 0 ) = 6 * 0^2 - 150
f ´( 0 ) = -150 ( Steigung negativ, fallend )

Zwischen -5 < x < 5 fällt die Funktion.
Bis x = - 5 steigend
Nach x = + 5 steigend

Frag nach bis alles klar ist.

Comments

Vielen Lieben Dank für Erklärung ich habe es so verstanden ich kann jetzt andere Aufgaben weiter bearbeiten! Dankeee und schönen Tag Ihnen noch!

---

Gern geschehen.
Bei Bedarf stell´auch weitere Aufgaben
ein.
Die Antworter werden es dir danken.

Answer 2

f '(x) <0

6x^2-150 <=

x^2 < 25

|x| < 5

L = (-5;5)

Comments

Ich hoffe Sie sind mir nicht böse aber könnten Sie mir erklären wie Sie darauf kommen das für die nächsten Aufgaben weiss wie vorzugehen ist! Viele Vielen Lieben dank für die Lösung!!

Answer 3

\(f(x)=2 x^{3}-150 x+5 \)
\(f´(x)=6 x^{2}-150  \)

\(6 x^{2}-150 =0→x^2=25 \)

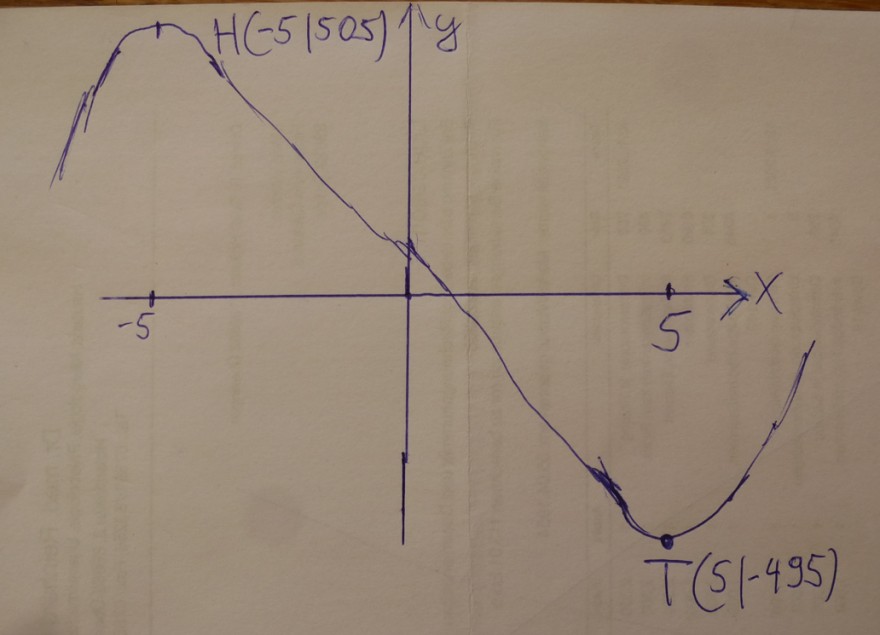
 \(x₁=5→f(5)=250-750+5=-495\)

Art des Extremwerts:

\(f´´(x)=12 x \)    \(f´´(5)=60>0  → Minimum \)

\(x₂=-5→f(-5)=2* (-5)^{3}-150 *(-5)+5=505\)

Art des Extremwerts:

\(f´´(x)=12 x \)    \(f´´(-5)=-60<0  →Maximum \)

Das Minimum liegt bei T(5|-495), und das Maximum liegt bei H(-5|505)

Im Intervall \({-5<x<5}\) ist \(f(x)\) streng monoton fallend.

Comments

Vielen Lieben Dank! ich habe alle erklärung mir zu herzen genommen und konnte jetzt viel lernen!!! Danke