Aufgabe:
…
Würde Hilfe bei dieser Aufgabe brauchen.. Wie gehe ich hier vor?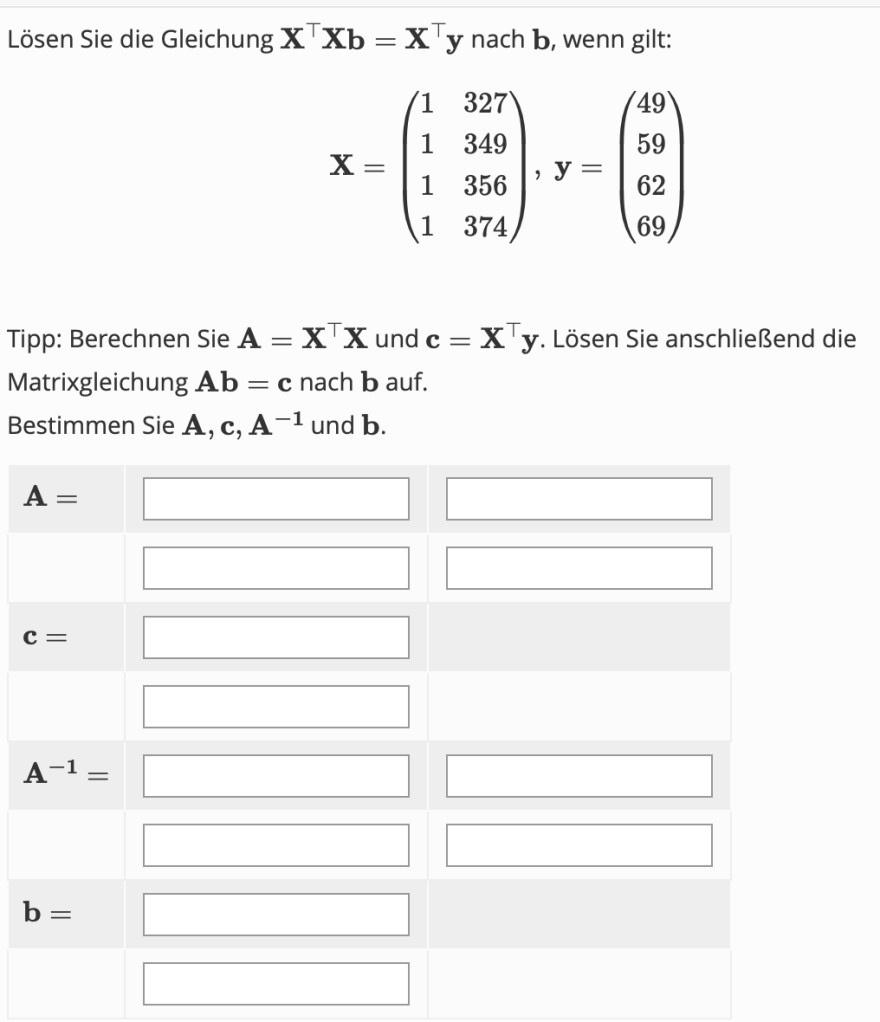
Text erkannt:
Lösen Sie die Gleichung \( \mathbf{X}^{\top} \mathbf{X} \mathbf{b}=\mathbf{X}^{\top} \mathbf{y} \) nach \( \mathbf{b} \), wenn gilt:
\( \mathbf{X}=\left(\begin{array}{ll} 1 & 327 \\ 1 & 349 \\ 1 & 356 \\ 1 & 374 \end{array}\right), \mathbf{y}=\left(\begin{array}{l} 49 \\ 59 \\ 62 \\ 69 \end{array}\right) \)
Tipp: Berechnen Sie \( \mathbf{A}=\mathbf{X}^{\top} \mathbf{X} \) und \( \mathbf{c}=\mathbf{X}^{\top} \mathbf{y} \). Lösen Sie anschließend die Matrixgleichung \( \mathbf{A} \mathbf{b}=\mathbf{c} \) nach \( \mathbf{b} \) auf.
Bestimmen Sie \( \mathbf{A}, \mathbf{c}, \mathbf{A}^{-1} \) und \( \mathbf{b} \).
\( \mathbf{A}= \)
\( \mathbf{c}= \)
\( \mathbf{A}^{-1}= \)
\( \mathbf{b}= \)
Problem/Ansatz: