Bestimmen Sie die folgenden Integrale

Question

Aufgabe: Bestimmen Sie die folgenden Integrale.

Text erkannt:

(b) \( \int \frac{1}{\sqrt{1+x^{2}}} \mathrm{~d} x \)

Tipp: Verwenden Sie in (b) die Substitution x = sinh t

Problem/Ansatz:

Ich habe die Lösung, verstehe sie aber nicht vollends.

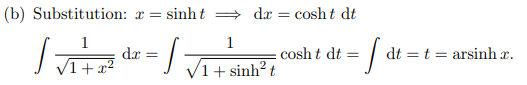
Text erkannt:

(b) Substitution: \( x=\sinh t \Longrightarrow \mathrm{d} x=\cosh t \mathrm{~d} t \)
\( \int \frac{1}{\sqrt{1+x^{2}}} \mathrm{~d} x=\int \frac{1}{\sqrt{1+\sinh ^{2} t}} \cosh t \mathrm{~d} t=\int \mathrm{d} t=t=\operatorname{arsinh} x \)

Ich verstehe den letzten Schritt quasi nicht. Wie kommen wir da auf dt=t=arsinh x? Der Anfang ist ja nur einsetzen.

Comments

Schau mal hier:

https://www.integralrechner.de/

Answer 1

Es ist nicht \(dt=t\), es ist \(\int dt=\int 1\,dt = t+C\), da eine Stammfunktion zu 1 eben \(t\) ist.

Und wenn \(x=\sinh t\) ist (Substitution, gibt den Zusammenhang zwischen \(t\) und \(x\)), so ergibt das durch Anwenden der Umkehrfunktion von \(\sinh\), die ist eben \(\rm arsinh\): \(t={\rm arsinh } x\).

Genau wie es auch bei \(x=\sin t\iff t =\arcsin x\) wäre.