Ups, tut mir leid. Passt es so?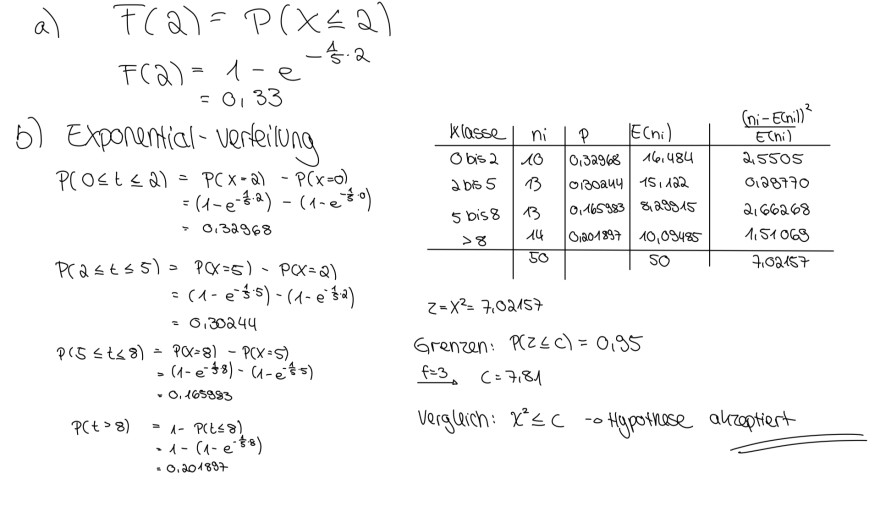
Text erkannt:
a)
\( \begin{aligned} F(2) & =P(x \leqslant 2) \\ F(2) & =1-e^{-\frac{4}{5} \cdot 2} \\ & =0,33 \end{aligned} \)
b) Exponential-verteilung
\( \begin{aligned} P(0 \leq t \leq 2) & =P(x-2)-P(x=0) \\ & =\left(1-e^{-\frac{1}{6} \cdot 2}\right)-\left(1-e^{-\frac{1}{3} \cdot 0}\right) \\ & =0,32968 \end{aligned} \)
\( \begin{aligned} P(\alpha \leq t \leq 5) & =P(x=5)-P(x=2) \\ & =\left(1-e^{-\frac{1}{5} \cdot 5}\right)-\left(1-e^{-\frac{1}{3}}\right) \\ & =0,30244 \\ P(5 \leq t \leq 8) & =P(x=8)-P(x=5) \\ & =\left(1-e^{-48}\right)-\left(1-e^{-\frac{1}{5}} 5\right) \\ & =0,165983 \end{aligned} \)
\( \begin{aligned} P(t>8)= & 1-P(t \leq 8) \\ & -1-\left(1-e^{-\frac{1}{8} 8}\right) \\ & =0.201897 \end{aligned} \)
\begin{tabular}{c|c|c|c|c}
Klasse & \( n_{i} \) & \( p \) & \( E\left(n_{i}\right) \) & \( \frac{\left(n_{i}-E\left(n_{i 1}\right)^{2}\right.}{E E n_{i}} \) \\
\hline 0 bis2 & 10 & 0,32968 & 16,484 & 2,5505 \\
2 b55 & 13 & 0130244 & 15,122 & 0,28770 \\
5 bis8 & 13 & 0,165983 & 8,29915 & 2,66268 \\
\( >8 \) & 14 & 0,201897 & 10,09485 & 1,51063 \\
\hline & 50 & & 50 & 7,02157
\end{tabular}
\( z=x^{2}=7,02157 \)
Grenzen: \( P(z \leqslant c)=0,95 \)
\( \xrightarrow{f=3} \quad c=7,81 \)
Vergleich: \( x^{2} \leq c \)-oHypothese akreptiert