Was ist die Dimension des Bildes?

Question

Aufgabe:

Problem/Ansatz:

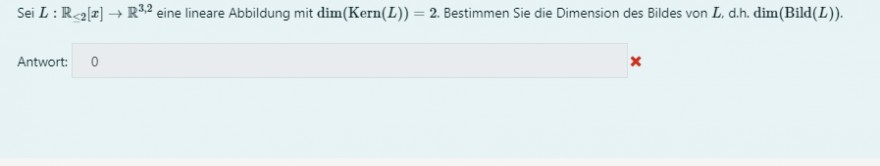
Text erkannt:

Sei $L: \mathbb{R}_{\leq 2}[x] \rightarrow \mathbb{R}^{3,2}$ eine lineare Abbildung mit $\operatorname{dim}(\operatorname{Kern}(L))=2$. Bestimmen Sie die Dimension des Bildes von $L$, d.h. $\operatorname{dim}(\operatorname{Bild}(L))$.
Antwort: 0

Wieso ist hier die richtige Antwort 1?

Answer 1

Laut Dimensionssatz gilt für lineare Abbildungen $\varphi: V\to W$

        $\dim V = \dim\operatorname{Kern}\varphi + \dim\operatorname{Bild}\varphi$

Comments

was war V noch mal?

---

$\varphi: V\to W$ sagt aus, dass $V$ der Definitionsbereich von $\varphi$ ist.

Answer 2

Hallo, nach Dimensionssatz gilt $\dim(\mathbb{R}_{\leq 2}[x])=\underbrace{\dim(\operatorname{Ker}(L))}_{=2}+\dim(\operatorname{Im}(L))$. Was ist $\dim(\mathbb{R}_{\leq 2}[x])$?