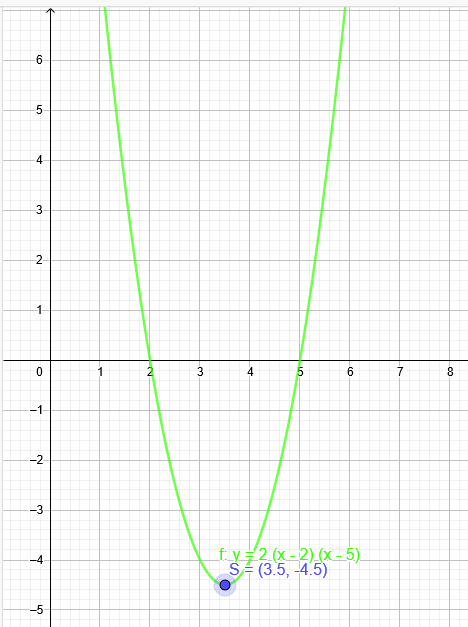
Gegeben sind die Nullstellen x₁ = 2 und x₂=5 sowie a=2. Stelle f(x) in Linearfaktordarstellung auf und bestimme den Scheitelpunkt S rechnerisch.
y=a*(x-N₁)*(x-N₂)
y=2*(x-2)*(x-5)
y=2x^2-14x+20|-20
y-20=2x^2-14x|:2
\( \frac{y-20}{2} \)=x^2-7x|+q.E.(\( \frac{-7}{2} \))^2=\( \frac{49}{4} \)
\( \frac{2y-40}{4} \)+\( \frac{49}{4} \)=x^2-7x+\( \frac{49}{4} \)
\( \frac{2y+9}{4} \)=(x-\( \frac{7}{2} \))^2|*4
2y+9=4*(x-\( \frac{7}{2} \))^2|-9
2y=4*(x-\( \frac{7}{2} \))^2-9|:2
y=2*(x-\( \frac{7}{2} \))^2-4,5
S(3,5|-4,5)