Homogenes DGL-System lösen, jedoch nicht diag.bar

Question

Text erkannt:

(ii) \( \begin{aligned} y_{1}^{\prime} & =y_{1}+6 y_{2}+3 y_{3} \\ y_{2}^{\prime} & =-2 y_{1}-6 y_{2}-2 y_{3} \\ y_{3}^{\prime} & =y_{1}+2 y_{2}-y_{3}\end{aligned} \)

Problem:

Ich weiß wie man vorgehen muss. Ich habe auch die Eigenvektoren ausgerechnet => nicht diagonalisierbar da aV = 3 und gV = 2. Ich brauche insgesamt 3 l.u abhängige Fkt. bzw Vektoren. Die ersten zwei konnte und wusste ich man die ausrechnet. Den dritten bekomme ich irgendwie nicht hin.

Ansatz:
Ich bin mit dem Ansatz
Yp(t)= (a1*t+b1, a1*t+b2, a3*t+b3)*e^(-2) an die Sache rangegangen. Am Anfang habe ich Yp'(t) gebildet und die Formel Yp(t) = A*Yp'(t) benutzt. Habe alles eingesetzt gekürzt und ordentlich aufgeschrieben. Koeffizientenvergleich habe ich versucht, aber ab da komme ich irgendwie trotzdem nicht weiter.
Könnte mir das jmd. bitte ausführlich aufschreiben, damit ich weiß wo ich einen Fehler mache. Danke im voraus

Answer 1

Hallo,

Du bekommst schließlich 6 Gleichungen mit 6 Variablen.(2 Gleichungen fallen weg, sind redundant)

Damit mußt Du freie Koeffizienten wählen und die restlichen Gleichungen durch Sie ausdrücken.

Ich habe erhalten:

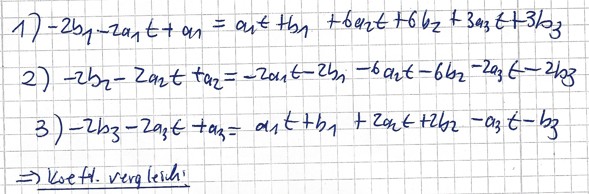
A) 0 = 3a₁ +6a₂ +3a₃

B) 0= 3b₁+6b₂+ 3b₃ -a₁

C) 0= -2a₁ -4a₂-2a₃ ->redundant

D) 0=-2b₁-4b₂-2b₂-a₂

E) 0= a₁ +2a₂+a₃   -->redundant

F) 0= b₁+2b₂ +b₃-a₃

_usw.

Ich verwende in solchen Fällen lieber die Hauptvektormethode (falls behandelt)

Sicher sind das dann möglicherweise andere Bezeichnungen als ich habe.

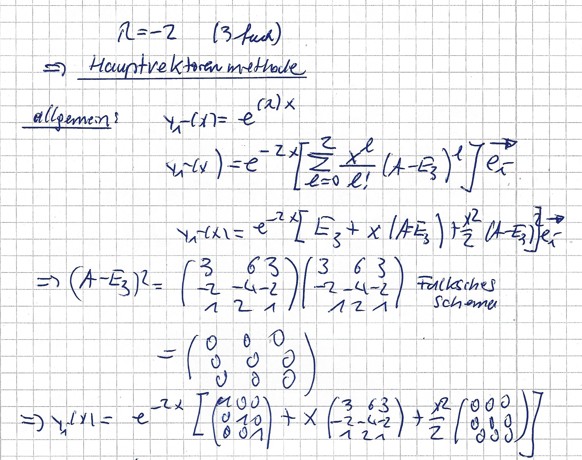
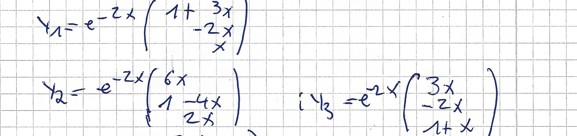
Kontrolle Wolfram Alpha:

\( \mathrm{y} 1(x)=c_{1} e^{-2 x}(3 x+1)+6 c_{2} e^{-2 x} x+3 c_{3} e^{-2 x} x \)

\( \mathrm{y} 2(x)=-2 c_{1} e^{-2 x} x-c_{2} e^{-2 x}(4 x-1)-2 c_{3} e^{-2 x} x \)
\( \mathrm{y} 3(x)=c_{1} e^{-2 x} x+2 c_{2} e^{-2 x} x+c_{3} e^{-2 x}(x+1) \)

Comments

Leider kenne ich die Hauptvektormethode nicht. Trotzdem habe ich alles nachvollziehen können. Dankeschön :)