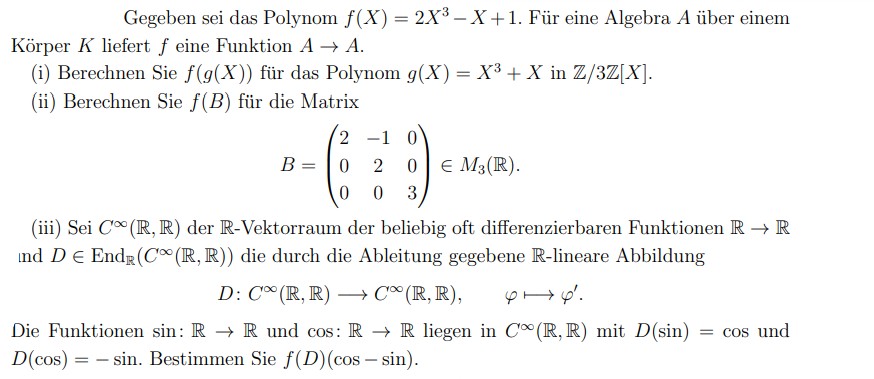
Text erkannt:
Gegeben sei das Polynom \( f(X)=2 X^{3}-X+1 \). Für eine Algebra \( A \) über einem Körper \( K \) liefert \( f \) eine Funktion \( A \rightarrow A \).
(i) Berechnen Sie \( f(g(X)) \) für das Polynom \( g(X)=X^{3}+X \) in \( \mathbb{Z} / 3 \mathbb{Z}[X] \).
(ii) Berechnen Sie \( f(B) \) für die Matrix
\( B=\left(\begin{array}{ccc} 2 & -1 & 0 \\ 0 & 2 & 0 \\ 0 & 0 & 3 \end{array}\right) \in M_{3}(\mathbb{R}) \)
(iii) Sei \( C^{\infty}(\mathbb{R}, \mathbb{R}) \) der \( \mathbb{R} \)-Vektorraum der beliebig oft differenzierbaren Funktionen \( \mathbb{R} \rightarrow \mathbb{R} \) ind \( D \in \operatorname{End}_{\mathbb{R}}\left(C^{\infty}(\mathbb{R}, \mathbb{R})\right) \) die durch die Ableitung gegebene \( \mathbb{R} \)-lineare Abbildung
\( D: C^{\infty}(\mathbb{R}, \mathbb{R}) \longrightarrow C^{\infty}(\mathbb{R}, \mathbb{R}), \quad \varphi \longmapsto \varphi^{\prime} . \)
Die Funktionen \( \sin : \mathbb{R} \rightarrow \mathbb{R} \) und \( \cos : \mathbb{R} \rightarrow \mathbb{R} \) liegen in \( C^{\infty}(\mathbb{R}, \mathbb{R}) \) mit \( D(\sin )=\cos \) und \( D(\cos )=-\sin \). Bestimmen Sie \( f(D)(\cos -\sin ) \).