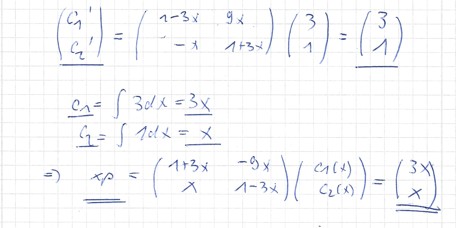Hallo,
deine partikuläre Lösung ist richtig.
Wolfram alpha bestätigt das.
https://www.wolframalpha.com/
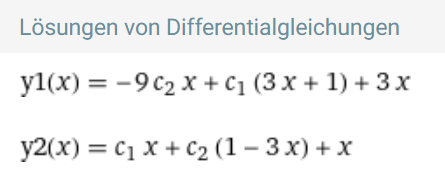
ich habe auch via Variation der Konstanten gerechnet , der Weg ist etwas anders:
Prinzipiell mußt Du das natürlich so rechnen, wie Ihr das hattet.
(habe kein Fehler gefunden)
Ich habe aber in der Aufgabe nicht speziell gelesen, welcher Weg genau vorgeschrieben wurde.