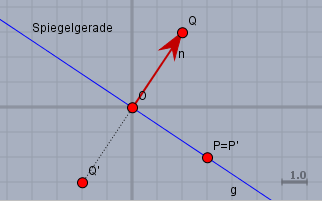
wenn Du die Spiegelmatrix \(A_S\) kennen würdest, so könntest Du mit ihr die Bildpunkte der Spiegelung berechnen. Du weißt, dass Punkte auf der Spiegelgeraden auf sich selbst abgebildet werden. So wie z.B. \(P=(3|-2)^T\) auf dem Bild
also ist
$$A_S \cdot \begin{pmatrix} 3 \\ -2 \end{pmatrix} = \begin{pmatrix} 3 \\ -2 \end{pmatrix}$$
Wohingegen ein Punkt \(Q\), der von Ursprung aus in Normalenrichtung der Spiegelgeraden verschoben wird, auf seine Negation abgebildet wird.
$$A_S \cdot \begin{pmatrix} 2 \\ 3 \end{pmatrix} = \begin{pmatrix} -2 \\ -3 \end{pmatrix}$$
Zusammengefasst kann man auch schreiben
$$A_S \cdot \begin{pmatrix} 3 & 2 \\ -2 & 3 \end{pmatrix} = \begin{pmatrix} 3 & -2\\ -2 & -3 \end{pmatrix}$$ bzw.:
$$\begin{aligned} A_S &= \begin{pmatrix} 3 & -2\\ -2 & -3 \end{pmatrix} \cdot \begin{pmatrix} 3 & 2 \\ -2 & 3 \end{pmatrix}^{-1} \\ &= \begin{pmatrix} 3 & -2\\ -2 & -3 \end{pmatrix} \cdot \begin{pmatrix} 3 & -2 \\ 2 & 3 \end{pmatrix} \frac{1}{13} \\ &= \frac{1}{13}\begin{pmatrix}5& -12\\ -12& -5\end{pmatrix}\end{aligned}$$
Noch ein Tipp: berechne mal die Eigenvektoren von \(A_S\).