Aufgabe:
Berechnen Sie den Grenzwert:
$$ \lim \limits_{x \rightarrow \infty}\left(x · \ln \left(\frac{x+1}{x-1}\right)\right) $$
Problem/Ansatz:
Ich habe zwei Ansätze: bei dem ersten bin mit nicht sicher, ob ich im letzten Schritt (x^2-1) im Nenner
so ableiten kann, wie ich es getan habe. Bei dem zweiten bin ich auf keine Lösung gekommen. Dort habe ich mit der Kettenregel den Term (x^2-1) im Nenner abgeleitet.
1.:
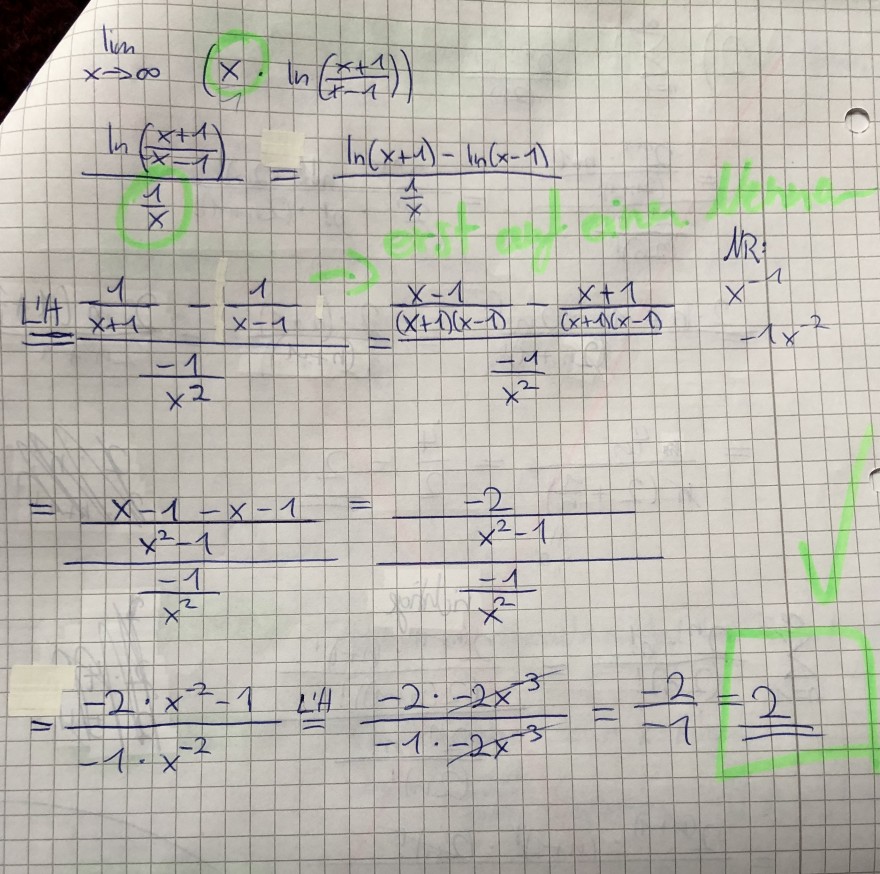
Text erkannt:
\( \lim \limits_{x \rightarrow \infty}\left(x \cdot \ln \left(\frac{x+1}{x-1}\right)\right) \)
$$ \frac{\ln \left(\frac{x+1}{x-1}\right)}{\frac{1}{x}}=\frac{\ln (x+1)-\ln (x-1)}{\frac{1}{x}} $$
\( \frac{\operatorname{Li} \frac{1}{x+1}-\frac{1}{x-1}}{\frac{-1}{x^{2}}}=\frac{\frac{x-1}{(x+1)(x-1)}-\frac{x+1}{(x+1)(x-1)}}{\frac{-1}{x^{2}}}-1 x^{-2} \)
\( =\frac{x-1-x-1}{\frac{x^{2}-1}{\frac{-1}{x^{2}}}}=\frac{-2}{\frac{-1}{x^{2}}} \)
\( =\frac{-2 \cdot x^{-2}-1}{-1 \cdot x^{-2}} \stackrel{L^{\prime} H}{=} \frac{-2 \cdot-2 x^{-3}}{-1 \cdot-2 x^{-3}}=\frac{-2}{-1}=2 \)
2.: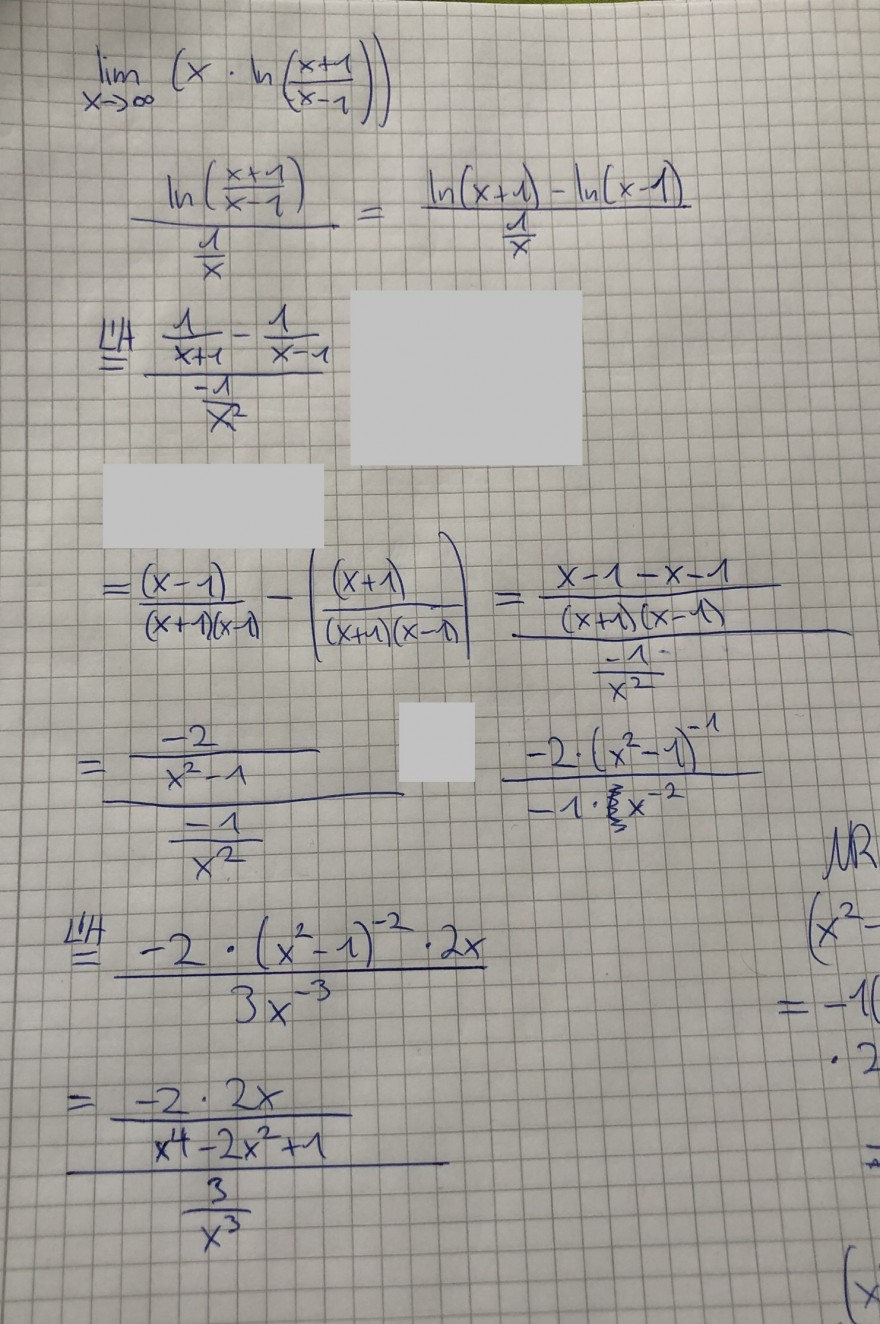
Text erkannt:
\( \lim \limits_{x \rightarrow \infty}\left(x \cdot \ln \left(\frac{x+1}{x-1}\right)\right) \)
\( \frac{\ln \left(\frac{x+1}{x-1}\right)}{\frac{1}{x}}=\frac{\ln (x+1)-\ln (x-1)}{\frac{1}{x}} \)
\( =\frac{\frac{1}{x+1}-\frac{1}{x-1}}{\frac{-1}{x^{2}}} \)
\( =\frac{(x-1)}{(x+1)(x-1)}-\left|\frac{(x+1)}{(x+1)(x-1)}\right|=\frac{x-1-x-1}{\frac{(x+1)(x-1)}{\frac{-1}{x^{2}}}} \)
\( =\frac{\frac{-2}{x^{2}-1}}{\frac{-1}{x^{2}}} \)
\( =\frac{-2 \cdot\left(x^{2}-1\right)^{-2} \cdot 2 x}{3 x^{-3}} \)
\( \frac{=-2 \cdot 2 x}{\frac{x^{4}-2 x^{2}+1}{\frac{3}{x^{3}}}} \)