Aufgabe: Fläche zwischen 2 Graphen Integrieren + Rotationsvolumen um X-Achse der Fläche
Problem/Ansatz:
Ich habe eine Aufgabe für die ich keine Lösung habe.
Es sollin Teil a) eine Fläche zwischen zwei Graphen Integriert werden und in Aufgabenteil b) dann diese Fläche um die X Achse rotieren und somit das Volumen des Körpers berechnet werden.
Ich würde mich freuen wenn jemand meine Rechnung kontrolieren könnte und mich auf meine Fehler aufmerksamm machen würde.
Falls möglich richtig vorrechnen.
Aufgabenstellung + Rechnung im Anhang.
obere Funktion: y2(x)=2π-x
untere Funktion: y1(x)=sin(2x)+1
in den Grenzen von [0,π]
Vielen Dank Leute !!!!
Gruß
Frost
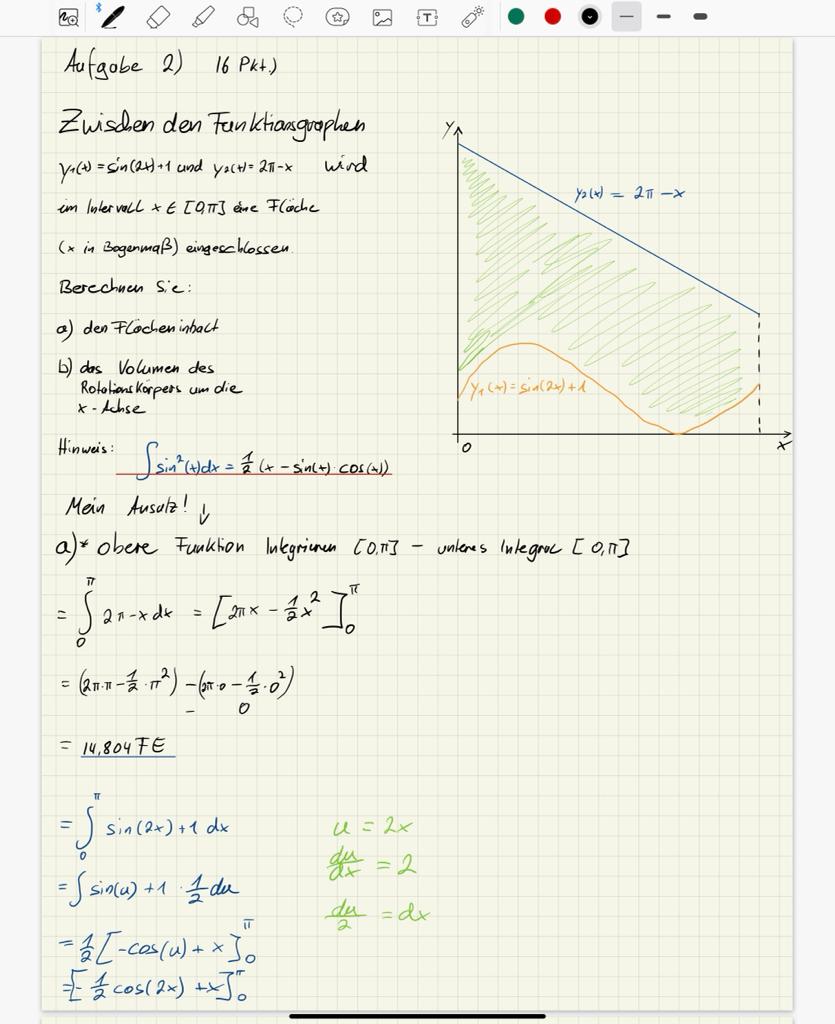
Text erkannt:
Au(gabe 2) 16 Pkt.)
Zwischen den Funktiansgraphen \( y_{1}(t)=\sin (2 t)+1 \) und \( y_{2}(t)=2 \pi-x \) wind im Intervall \( +\in[0, \pi] \) eire \( F \) loche \( (x \) in Bogenma \( \beta \) ) eingesehlossen.
Berechnen Sie:
a) den Flochenintact
b) das Volumen des Rotolias koopers um die
Hinweis: \( \int \sin ^{2}(t) d x=\frac{1}{2}(x-\sin (t) \cos (t)) \)
Mein Ausulz! b
a)* Obere Funktion Integrimen \( [0, \pi] \) - unteres integroe \( [0, \pi] \)
\( =\int \limits_{0}^{\pi} 2 \pi-x d x=\left[2 \pi x-\frac{1}{2} x^{2}\right]_{0}^{\pi} \)
\( \begin{array}{c} =\left(2 \pi \cdot \pi-\frac{1}{2} \cdot \pi^{2}\right)-\left(2 \pi \cdot 0-\frac{1}{2} \cdot 0^{2}\right) \\ -0 \end{array} \)
\( =14,804 F E \)
\( \begin{array}{ll} =\int \limits_{0}^{\pi} \sin (2 x)+1 d x & u=2 x \\ =\int \limits_{\sin (u)+1} \frac{1}{2} d u & d u \\ =\frac{1}{2}[-\cos (u)+x]_{0}^{\pi} & \frac{d u}{2}=2 \\ =\left[-\frac{1}{2} \cos (2 x)+x\right]_{0}^{\pi} & \end{array} \)

Text erkannt:
\( \begin{array}{l} =\left(\frac{-\cos (2 \pi)}{2}+\pi\right)-\left(\frac{-\cos (20)}{2}+0\right) \\ =\pi \\ \text { Flache }=14,804-\pi \\ =11,66 \mp E \end{array} \)
b)
\( V=\pi \cdot \int \limits_{a}^{b}(f(x))^{2} d x \)
Beide Fanklionen gleidsetzen:
\( \begin{aligned} \sin (2 x)+1 &=2 \pi-x \quad 1-2 \pi+x \\ \sin (2 x)-5,28+x &=0 \end{aligned} \)
Funktion in Formel einsetzen
\( V=\pi \cdot \int \limits_{0}^{\pi}(\sin (2 x)-5,28+x)^{2} d x \)
Funkton Quadrienen! Hilfsinlegral aus Aerfgoberstllang fair
\( \begin{aligned} \int \sin ^{2}(x) d x &=\frac{1}{2}(x-\sin (t) \cdot \cos (t)) \\ &\left.=\frac{1}{2} \cdot(x-\sin (2 t)) \cos (2 x)\right) \end{aligned} \)

Text erkannt:
\( \pi \cdot\left(\frac{1}{2}(x-\sin (2 t) \cdot \cos (2 x))+\int(-5,28+x)^{2} d x\right) \)
\( (-5,28+t) \cdot(-5,28+x) \)
\( =27,88-10,56 x+x^{2} \)
\( \pi \cdot\left(\frac{1}{2} \underset{\text { Farkgis Teil IntGaral }}{ }(x-\sin (2 x) \cdot \cos (2 x))+\int 27,88-10,56 x+x^{2} d x\right. \)
\( =\pi \cdot\left[\frac{1}{2}(x-\sin (2 t) \cdot \cos (2 x))+27,88 x-5,28 x^{2}+\frac{1}{3} x^{3}\right]_{0}^{\pi} \)
obere Grenze - untere Grenze
\( \begin{array}{l} \left.=\pi \cdot\left[\frac{1}{2} \cdot(\pi-\sin (2 \cdot \pi)) \cdot \cos (2 \cdot \pi)\right)+27,88 \cdot \pi-5,28 \cdot \pi^{2}+\frac{1}{3} \cdot \pi^{3}\right]-0 \\ \underline{V=148,856 V E} \end{array} \)
Orline Reducr sogt \( V=138,97 \mathrm{VE} \) moglicherweise Reduct er midt in Bogenma/ B?!