Zwei Matrizen sind ähnlich, falls es eine invertierbare Matrix P gibt mit B=PAP-1.
1. Hier darfst du als Einträge alle reellen Zahlen wählen, du musst also eine Matrix P finden, die die obige Eigenschaft erfüllt:
Es gilt
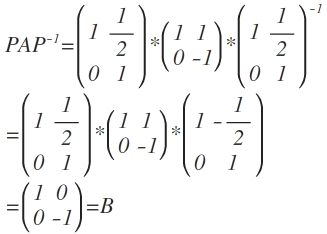
PA{ P }^{ -1 }=\begin{pmatrix} 1 & \frac { 1 }{ 2 } \\ 0 & 1 \end{pmatrix}*\begin{pmatrix} 1 & 1 \\ 0 & -1 \end{pmatrix}*{ \begin{pmatrix} 1 & \frac { 1 }{ 2 } \\ 0 & 1 \end{pmatrix} }^{ -1 }\\ =\begin{pmatrix} 1 & \frac { 1 }{ 2 } \\ 0 & 1 \end{pmatrix}*\begin{pmatrix} 1 & 1 \\ 0 & -1 \end{pmatrix}*{ \begin{pmatrix} 1 & -\frac { 1 }{ 2 } \\ 0 & 1 \end{pmatrix} }\\ =\begin{pmatrix} 1 & 0 \\ 0 & -1 \end{pmatrix}=B
Also sind A und B ähnlich, wenn K=IR.
2. Wenn K=F2={0;1}, dürfen die Einträge der Matrix P nur aus 0 und 1 bestehen. Hierbei gibt es keine Matrix P, die die Gleichung B=PAP-1 erfüllt (das kannst du einfach ausprobieren, so viele Möglichkeiten gibt es da nicht, wie P aussieht). A und B sind also nicht ähnlich, wenn K=F2={0;1}.