Folgen und Reihen | Konvergenz, Divergenz, Nullfolge

Question

Es seien C,D ∈ ℝ. Nun soll ich zeigen, dass die Folge konvergiert:

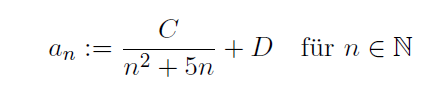
Text erkannt:

\( a_{n}:=\frac{C}{n^{2}+5 n}+D \quad \) für \( n \in \mathbb{N} \)

Um dies zu zeigen soll ich diese Definition verwenden:

Eine Folge (a_n)_n∈ℕ reeller zahlen heißt konvergent mit Grenzwert a ∈ ℝ, falls gilt:

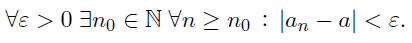
Text erkannt:

\( \forall \varepsilon>0 \exists n_{0} \in \mathbb{N} \forall n \geq n_{0}:\left|a_{n}-a\right|<\varepsilon \)

Notation:

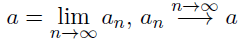
Ich weiß hier nicht genau wie ich anzufangen habe, kann mir da jemand helfen?

Danke!

Text erkannt:

\( a=\lim \limits_{n \rightarrow \infty} a_{n}, a_{n} \stackrel{n \rightarrow \infty}{\longrightarrow} a \)

Comments

Habe soeben dieselbe(?) Frage auf der mathelounge bei Leidensgenossen von dir gelesen. Denen war auch völlig schleierhaft, wie sich das D mit einem "kleinen" Epsilon überhaupt verträgt.

Vielleicht findest du den entsprechenden Fragetext wieder. Nur so viel. Wenn der Grenzwert z.B. D wäre, dann kommt in der Rechnung z.B. | (D+Epsilon) - D| vor. Diese Differenz zwischen den Betragsstrichen geht, wenn Epsilon gegen 0 geht, dann schon gegen 0. Du musst das einfach formal sauber aufschreiben.

Answer 1

Hallo

ist dir klar , was der GW ist? dann bestimme doch einfach ein N(ε) so dass für alle n>N |an-GW|<ε, dabei muss N nicht das kleinste mögliche sein, so dass man auch grob abschätzen kann. so ist etwa 1/(2n^2+n)<1/(2n^2)

Gruß lul

Comments

Bin etwas überfordert... tut mir leid

---

bzw. was passiert mit C und D, und wo ist die 5 hin?

Durch kehrwerte?

---

hab es nicht geschafft..

---

beim verstehen von der Definition hab ich schon meine probleme

---

Hallo

1, dass für n->oo der Bruch gegen 0 geht siehst du doch hoffentlich? als GW ist D

jetzt musst du ein n suchen, so dass |an-D|=|C|/(2n^2+5n)<ε

also 2n^2+5n>|C|/ε  daraus 2n^2>|C|/ε. und jetzt kannst du hoffentlich N  bestimmen?

lul