Analysis. Kurvendiskussion von f ( x ) = - 1/256 * ( x + 4 * t ) * x^3 - t

Question

Hallo alle zusammen. Übe gerade für Mathe und komme bei diesem Blatt nicht weiter. Wenn mir jemand helfen könnte, wäre ich sehr dankbar. :) Rechenschritte würden mir helfen.

Comments

Welche Aufgabe ??

---

Eig. alle : / . Aber ab 1.3 wäre am hilfreichsten. Die ersten 2 (1.1 / 1.2) bin ich grad selber am Knobeln. Aber ab 1.3 ist vorbei :( . 

---

Wobei hast denn bei 1.3 genau Schwierigkeiten. Die Bedingung für ein Extrempunkt kennst du doch oder?

Also rechne ihn doch mal aus.

Answer 1

f ( x ) = - 1/256 * ( x + 4 * t ) * x^3 - t

f3 ( x ) =  - 1/256 * ( x + 4 * 3 ) * x^3 - 3
f3 ( x ) =  - 1/256 * ( x + 12 ) * x^3 - 3
f3 ( x ) =  - 1/256 * ( x^4 + 12 * x^3 ) - 3

f3 ´( x ) =  -1/256 * ( 4 * x^3 + 36 * x^2 )
Stellen mit waagerechter Tangente
( 4 * x^3 + 36 * x^2 ) = 0
x^2 * ( 4 * x + 36 ) = 0
Satz vom Nullprodukt
x^2 = 0
x = 0
und
4 * x + 36 = 0
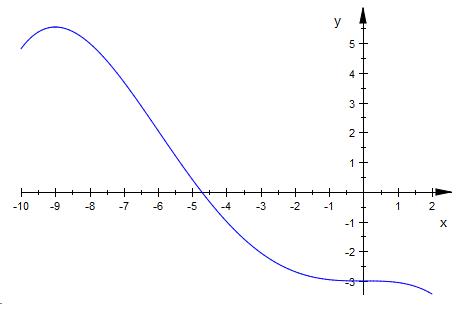
x = -9
f3 ´´ ( x ) =  -1/256 * ( 12 * x^2 + 72 * x )
f3 ´´ ( 0 ) =  -1/256 * ( 12 * 0 + 72 * 0 ) = 0
f3 ´´ ( -9 ) =  -1/256 * ( 12 * (-9)^2 + 72 * (-9) ) =-1.26 ( Hochpunkt )
Monotonie > 0
-1/256 * ( 4 * x^3 + 36 * x^2 )
4 * x^3 + 36 * x^2 < 0
x^2 * ( 4 * x + 36 ) < 0
x^2 ist stehts > 0
4 * x + 36 < 0
x < -9
bei x = 0 findet kein Wechsel der Monotonie statt.
x = 0 ist kein Extrempunkt.

Comments

f3 ´´ ( x ) =  -1/256 * ( 12 * x^2 + 72 * x )
Wendepunkte
-1/256 * ( 12 * x^2 + 72 * x )  = 0
x * ( 12 * x + 72 ) = 0
x = 0
und
12 * x + 72 = 0
x = -6

Rechtskrümmung
-1/256 * ( 12 * x^2 + 72 * x )  < 0
-1/256 * 12 * x * ( x + 6 ) < 0
- x * ( x + 6 ) < 0
x * ( x^+ 6 ) > 0

1 Fall
x > 0 und  x + 6 > 0 
x > 0

2 Fall
x < 0 und  x + 6 < 0 
x < 0 und x < -6
also
x < -6

Rechtskrümmung
minus unendlich bis -6
0 bis unendlcih

---

f ( x ) = - 1/256 * ( x + 4 * t ) * x³ - t

Schnittpunkt für alle t
- 1/256 * ( x + 4 * t1 ) * x³ - t1 = - 1/256 * ( x + 4 * t2 ) * x³ - t2
- 1/256 * ( x + 4 * t1 ) * x³ - (- 1/256 * ( x + 4 * t2 ) * x³ ) = - t2 + t1
- 1/256 * x^3 * ( ( x + 4 * t1 )  - ( x + 4 * t2 )  )  = t1 - t2
- 1/256 * x^3 * ( x + 4 * t1   - x - 4 * t2   )  = t1 - t2
- 1/256 * x^3 * ( 4 * t1  - 4 * t2   )  = t1 - t2
- 1/256 * x^3 * 4 * ( t1  - t2   )  = t1 - t2  | : ( t1 - t2 )
- 1/256 * x^3 * 4 =  1
x  = -4

Bei x = -4 schneiden sich alle Kurven der Funktionsschar.

---

f ( x ) = - 1/256 * ( x + 4 * t ) * x³ - t
f ( x ) = - 1/256 * ( x^4 + 4 * t * x^3 ) - t
f ´( x ) =  - 1/256 * ( 4 * x^3 + 12 * t * x^2 )
4 * x^3 + 12 * t * x^2 = 0
x^2 * ( 4 * x + 12 * t ) = 0
x = 0
und
4 * x + 12 * t = 0
x = -4
4 * -4 + 12 * t = 0
12 * t = 16
t = 16 / 12 = 4 / 3

Für t = 4/3 ist bei x = -4 ein Extrempunkt vorhanden.

So. Keine Lust mehr.

---

1.3.4.
Der höchste erreichbare Punkt ist der linke Extremwert
f ´( x ) =  - 1/256 * ( 4 * x³ + 12 * t * x² )
- 1/256 * ( 4 * x³ + 12 * t * x² )  = 0
x = 0
und
4 * x + 12 * t = 0
x = - 3 * t
f ( x ) = - 1/256 * ( x + 4 * t ) * x³ - t
f ( x ) = - 1/256 * ( (-3)*t + 4 * t ) * (-3*t)^3 - t  = 23
27*t^4 / 256 - t = 23
Durch raten
t = 4
Alle Funktionen mit t <= 4 liegen im Wertebereich <= 23.