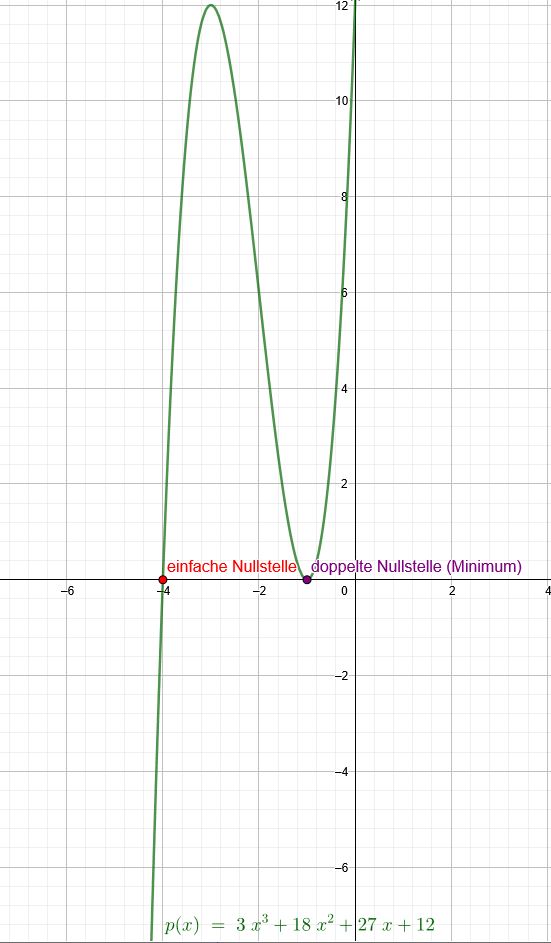
\( P_{2}(x)=3 x^{3}+18 x^{2}+27 x+12 \) \( x=-1 \) ist eine Nullstelle
Polynomdivision:
\( (3 x^{3}+18 x^{2}+27 x+12):(x+1) =3x^2+15x+12\)
\(3x^2+15x+12=0\)
\(x^2+5x+4=0 |-4\)
\(x^2+5x=-4\)
\((x+\frac{5}{2})^2=-4+(\frac{5}{2})^2=-4+6,25=2,25 |\sqrt{~~} \)
1.)
\(x+2,5=1,5 \)
\(x_1=-1 \)
2.)
\(x+2,5=-1,5 \)
\(x_2=-4 \)
\( P_{2}(x)=3 x^{3}+18 x^{2}+27 x+12=\red{3}*( x^{3}+6 x^{2}+9 x+4) \)
\( P_{2}(x)=\red{3}*(x+1)*(x+1)*(x+4)=\red{3}*(x+1)^2*(x+4) \)
\(x=-1\) ist eine doppelte Nullstelle (Extremwert)
\(x=-4\) ist eine einfache Nullstelle