- 2 = x•\( \sqrt{x} \) = x *\( x^{0,5} \)=\( x^{1,5} \)
x = \( \sqrt[1,5]{-2} \)= \( -2^{1,5} \) → komplexe Zahl (Wolfram)
Oder so:
- 2 = x•\( \sqrt{x} \) = \( \sqrt{x^2• x} \) = \( \sqrt{x^3} \)
\( \sqrt{x^3} \) = - 2
\( \sqrt{x^3} \) + 2= 0 → Es existiert keine Lösung ∈ ℝ.
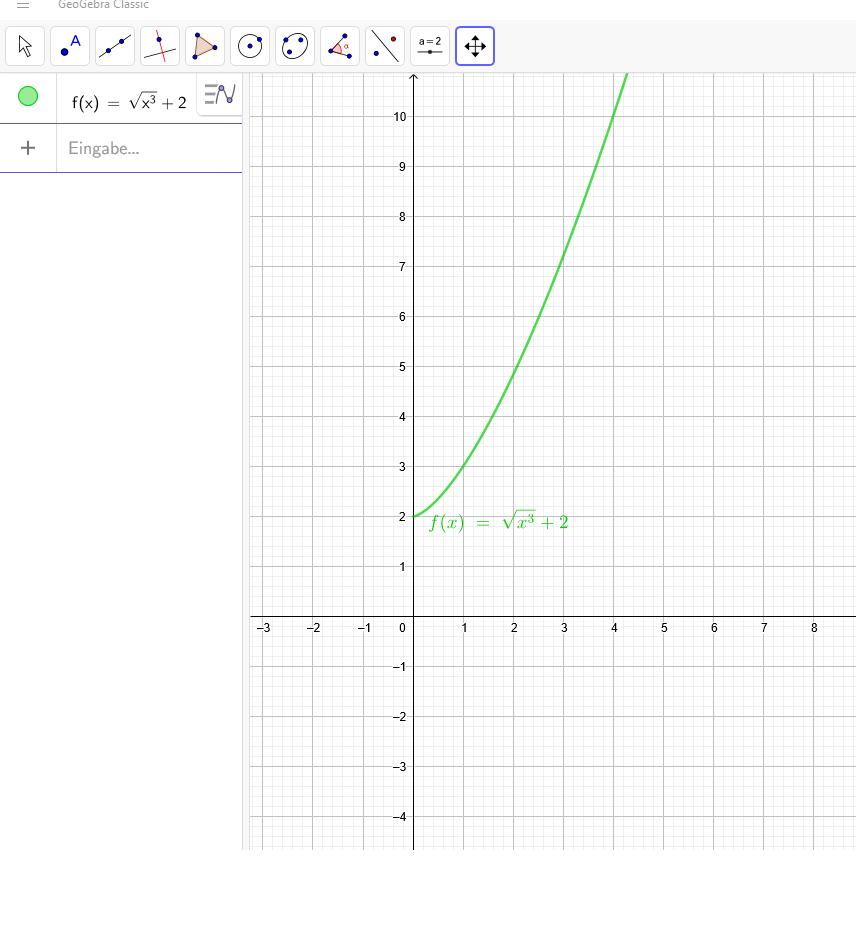
Text erkannt:
\( f(x)=\sqrt{x^{3}}+2=\Omega \)
Eingabe...