Bestimmen Sie die Matrix X und die Determinante von X.

Question

Aufgabe:

Gegeben sei die Matrixgleichung X⋅A+X⋅B=C mit den Matrizen

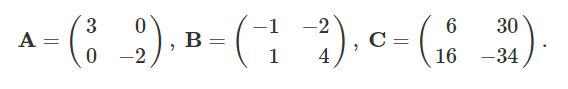
Text erkannt:

$\mathbf{A}=\left(\begin{array}{rr}3 & 0 \\ 0 & -2\end{array}\right), \mathbf{B}=\left(\begin{array}{rr}-1 & -2 \\ 1 & 4\end{array}\right), \mathbf{C}=\left(\begin{array}{rr}6 & 30 \\ 16 & -34\end{array}\right)$

Bestimmen Sie die Matrix X und die Determinante von X.

Problem/Ansatz:

Bei meinem Rechenweg scheint ein Fehler drinn zu sein. Ich habe folgendermaßen gerechnet:

Gleichung nach x aufgelöst: x= C*(A+B)^-1

Dann die Matrixen eingesetzt. Für x kam dann die Matrixe:

x=(2, 10, -2,66, -11,33).

Für die Determinante von x habe ich folgendeermaßen gerechnet: (2*(-11,33))- (10*(-2,66))= 3,94

Könnte mir vielleicht jemand sagen wo ich falsch gerechnet habe? Bzw. den Rechenweg/Lösung online stellen? Vielen Dank im Voraus

Answer 1

Aloha :)

Willkommen in der Mathelounge... \o/

Gegeben sind die Matrizen:$$A=\left(\begin{array}{rr}3 & 0\\0 & -2\end{array}\right)\quad;\quad B=\left(\begin{array}{rr}-1 & -2\\1 & 4\end{array}\right)\quad;\quad C=\left(\begin{array}{rr}6 & 30\\16 & -34\end{array}\right)$$

Dafür sollen wir die folgende Gleichung lösen:$$\left.X\cdot A+X\cdot B=C\quad\right|\text{\(X\) ausklammern}$$$$\left.X\cdot(A+B)=C\quad\right|\cdot(A+B)^{-1} \text{ von rechts}$$$$\left.X=C\cdot(A+B)^{-1}\quad\right|\cdot(A+B)^{-1} \text{ von rechts}$$

Wir setzen ein:$$X=\left(\begin{array}{rr}6 & 30\\16 & -34\end{array}\right)\cdot\left(\left(\begin{array}{rr}3 & 0\\0 & -2\end{array}\right)+\left(\begin{array}{rr}-1 & -2\\1 & 4\end{array}\right)\right)^{-1}$$$$\phantom{X}=\left(\begin{array}{rr}6 & 30\\16 & -34\end{array}\right)\cdot\left(\begin{array}{rr}2 & -2\\1 & 2\end{array}\right)^{-1}=\left(\begin{array}{rr}6 & 30\\16 & -34\end{array}\right)\cdot\left(\begin{array}{rr}\frac13 & \frac13\\[1ex]-\frac16 & \frac13\end{array}\right)$$$$\phantom{X}=\left(\begin{array}{rr}-3 & 12\\11 & -6\end{array}\right)$$

Die Determinante dieser Matrix ist:$$\operatorname{det}(X)=(-3)\cdot(-6)-11\cdot12=-114$$

Comments

Aloha my friend! Vielen Lieben Dank! Nach deinem Rechenweg habe ich es sehr gut verstanden!

Grüße

---

Hallo kurze Frage, wie kommt man hier auf die Brüche in der vorletzten Zeile ganz am Ende. MFG

---

Da wurde die inverse Matrix zu $\begin{pmatrix}2 & -2\\1 & 2\end{pmatrix}$ gebildet.

---

Hallo , ich habe eine sehr ähnliche Aufgabe, bekommen aber immer das Falsche Ergebnis, könntest du mi helfen?

Text erkannt:

Gegeben sei die Matrixgleichung $\mathbf{A} \cdot \mathbf{X}+\mathbf{B} \cdot \mathbf{X}=\mathbf{C}$ mit den Matrizen
$\mathbf{A}=\left(\begin{array}{ll} 2 & 3 \\ 3 & 3 \end{array}\right), \mathbf{B}=\left(\begin{array}{rr} 1 & -3 \\ -5 & -1 \end{array}\right), \mathbf{C}=\left(\begin{array}{rr} 27 & 21 \\ -32 & -16 \end{array}\right)$
Bestimmen Sie die Matrix $\mathbf{X}$ und ihre Determinante.
\begin{tabular}{|l|l|l|}
\hline $\mathbf{X}=$ & $16.0$ & $10.5$ \\
\hline$-16.0$ & $-8.0$ \\
\hline $\operatorname{det} \mathbf{X}=$ & $40.0$ & \\
\hline
\end{tabular}

Habe nur 0,2 von 1 Punkt bekommen. MFG

---

@Tschakabumba könntest du mir helfen meinem Fehler auf die schliche zu kommen?

---

@maxmillion2001:

Du hast übersehen, dass die Matrix $X$ bei dir von rechts multipliziert wird, in der Original-Frage wird die Matrix aber von links multipliziert. Das ist ein kleiner Unterschied, denn:$$A\cdot X+B\cdot X=C$$$$(A+B)\cdot X=C$$$$X=(A+B)^{-1}\cdot C$$Das heißt, du musst die inverse Matrix von links an $C$ multiplizieren.

Das sieht für dich konkret so aus:$$A+B=\begin{pmatrix}3 & 0\\ -2 & 2\end{pmatrix}$$Das musst du invertieren:$$(A+B)^{-1}=\begin{pmatrix}\frac13 & 0\\[1ex]\frac13 & \frac12\end{pmatrix}$$und dann von links an die Matrix $C$ multiplizieren:

$$X=(A+B)^{-1}\cdot C=\begin{pmatrix}9 & 7\\-7 & -1\end{pmatrix}$$

Die Determinante von $X$ beträgt:$$\operatorname{det}(X)=-9\cdot1+7\cdot7=40$$

Die Determinante war richtig, deswegen gab es wohl 0,2 Punkte.

---

Vielen Dank, das hat mir sehr geholfen.

---

@Tschakabumba könntest du mir noch einmal kurz weiterhelfen bei folgender Problematik:

Text erkannt:

Lösen Sie die Gleichung $\mathbf{X}^{\top} \mathbf{X} \mathbf{b}=\mathbf{X}^{\top} \mathbf{y}$ nach $\mathbf{b}$, wenn gilt:
$\mathbf{X}=\left(\begin{array}{ll} 1 & 378 \\ 1 & 381 \\ 1 & 401 \\ 1 & 438 \end{array}\right), \mathbf{y}=\left(\begin{array}{l} 63 \\ 68 \\ 72 \\ 75 \end{array}\right)$
Tipp: Berechnen Sie $\mathbf{A}=\mathbf{X}^{\top} \mathbf{X}$ und $\mathbf{c}=\mathbf{X}^{\top} \mathbf{y}$. Lösen Sie anschließend die Matrixgleichung $\mathbf{A b}=\mathbf{c}$ nach $\mathbf{b}$ auf. Bestimmen Sie $\mathbf{A}, \mathbf{c}, \mathbf{A}^{-\mathbf{1}}$ und $\mathbf{b}$.
$\mathbf{A}=$
$\mathbf{c}=$
$\mathbf{A}^{-\mathbf{1}}=$
$\mathbf{b}=$

Vielen Dank im vorhinein.